Galileo, died 1642

Physics History A Timeline 'Contributor' Names with Birth and Death Dates names in order by birth date with brief notes on their contributions

Isaac Newton, born 1642

Home page > RefInfo menu >

Math-Science topics menu >

This Physics History (Timeline) page

! Note !
The bottom part of this page is 'unfinished'.
Links were being added and tested in 2017.
To be continued.
Biographical notes are to be added, and
physicist names and dates may be added.

Links to start of physicist
(and applied mathematician)
birthdays below :

500 BCE     0 BCE     500    

1000     1200     1400     1500     1600    

CONSTRUCTION-SITE    

1700     1800     1900    

INTRODUCTION : This page presents a 'timeline' of the History of Physics (Wikipedia link). This page contains physicist names and their contributions --- along with their birth and death dates --- with 'external' links to more information on each physicist. We start from the oldest 'contributors' at the top of the page to the more recent contributors at the bottom of the page. The names are ordered according to their birth year (or an estimate thereof). This is to keep together the physicists who received their early training at about the same time (usually in their teens). Hence they were likely to be contemporories who would cooperate and/or compete with each other. And they were likely to be building on a common set of work and teaching --- in particular, the teaching and work of others born a decade or two before them. SOURCES AND SUBJECTS OF THIS WEB PAGE : I started this web page in 2017 based on a Math History/Timeline page that I started around 2011. That web page traces many of the outstanding mathematical contributions throughout human history --- and provides birth and death dates, along with brief biographical notes, for many of the contributors to the body mathematical. Many of the early mathematicians on that page were also 'physicists' --- in the sense that they were 'astronomers' or 'cosmologists' or 'star cartographers' ---- and/or they wrote on the subjects of 'optics' or 'hydraulics' or 'mechanics' or 'statics' --- and simple machines such as levers and pulleys and linkages. In the study of time and astronomy, the 'ancients' used tools such as the gnomon, the cross-staff, the backstaff, the quadrant, the astrolabe, the sextant, and the orrery. The subject of 'mechanical dynamics' really took off around the time of Galileo, Huygens, and Newton --- around the 1600's. And it wasn't until around 1900 that the subjects of 'atomic physics', 'nuclear physics', and 'quantum mechanics' began to be developed. 'Classical mechanics' has come to include a wide variety of categories. And in 'general physics' there are a variety of concepts. --- For each of the physicists on this page, I provide a web link for further information on the individual and his/her discoveries. Most of the links are to the Wikipedia web site. When Wikipedia does not have a page for the physicist, I may provide a link to a 'home page' of the physicist --- or a link to a web search on that physicist's name. If the list on this page does not satisfy, you may wish to try the Lists of physicists at Wikipedia --- ordered alphabetically, by last name. For other orderings, here is a Wikipedia page for physicists by nationality, and here is a Wikipedia page for physicists by century --- which are ordered alphabetically in those groupings. And here is a Wikipedia page named List of theoretical physicists. More physicists can be found via the Galileo Project of Rice University. Another list of physicists is available at thefamouspeople.com. Or, you can try a WEB SEARCH on the keywords list of physicists. This Should I become a mathematician? thread at physicsforums.com provides many interesting source books on mathematics and mathematicians --- and physics and physicists. For a compact list of physicists names --- with birth and death years, see Mark Manley's list at Kent State University. (Like most pages in userid directories at universities, this page is likely to go dead/missing within a decade. It was updated in 2017.) SEARCHING THIS PAGE : If you are looking for some particular information, you can use the text search function of your web browser. For example, if you are looking for information on 'quantum mechanics' or 'celestial mechanics' or 'statistical mechanics', enter a keyword such as 'quantum', 'celestial', or 'statistic' in the text search entry field of your web browser. Some other key-strings to try: 'magnet', 'electr', 'optic', 'light', 'atom', 'mole', 'prot', 'neut', 'mass', 'acceleration', 'energy', 'force', ... THE WONDERMENT OF IT ALL : Physics offers many occasions for wonderment at the logical and geometric beauties of that subject matter --- and wonderment at some of the nobler accomplishments that are possible from the minds of humans. The development of physics knowledge over the centuries is an amazing human achievement. It is one of the 'purer' pursuits of man. Physics, like art, provides galleries of wondrous creations (or discoveries) --- galleries based on subjects such as astronomy, optics, hydraulics, force and mass balance, dynamics, orbits, fields, particles, waves, etc. etc. Enjoy the way physics history ('natural philosophy') has unfolded!

START of PHYSICS TIMELINE :

Eudoxus of Cnidus (ca. 408 to 355 B.C.) was a Greek astronomer, mathematician, scholar and student of Plato. Eudoxus is considered by some to be the greatest of classical Greek mathematicians, and in all antiquity, second only to Archimedes. He rigorously developed Antiphon's method of exhaustion, a precursor to the integral calculus which was also used in a masterly way by Archimedes in the following century. In applying the method, Eudoxus proved such mathematical statements as: areas of circles are to one another as the squares of their radii, volumes of spheres are to one another as the cubes of their radii, the volume of a pyramid is one-third the volume of a prism with the same base and altitude, and the volume of a cone is one-third that of the corresponding cylinder. Aristarchus of Samos (ca. 310 to 230 B.C.) was a Greek mathematician and astronomer who presented the first known model of the solar system that placed the Sun at the center of the known universe with the Earth revolving around it. Archimedes (287 to 212 B.C.) was a Greek mathematician, physicist (before the word was used), engineer, inventor, and astronomer. He showed many surprising geometric relationships, such as: For a sphere inside a minimal containing cylinder, the surface area of the sphere is the same as that of the cylinder. He also made scientific discoveries concerning centers-of-gravity and floating bodies. He approximated the value of pi via inscribed and circumscribed regular polygons about a circle. He devised war engines to repel Romans who besieged Syracuse, Greece. The Romans eventually took the city and sent soldiers to get Archimedes, to meet with the Roman governor. Archimedes, who often drew figures in the sand, told the soldiers "Don't disturb my circles!" (probably in Greek, eh?). When he ("the Sand Reckoner") tried to stop the soldiers, they killed him, perhaps thinking that he was attacking (or insulting) them. (This is one of the many interesting tales of the deaths of physicists.) Eratosthenes of Cyrene (ca. 276 to 195 B.C.) Greek mathematician, geographer, poet, astronomer, and music theorist. He invented a system of latitude and longitude. He was the first person to calculate the circumference of the earth (apparently believed the earth was a sphere, not flat). He also proposed a simple algorithm for finding prime numbers. This algorithm is known in mathematics as the 'Sieve of Eratosthenes'. The sieve of Eratosthenes is one of a number of prime number sieves. Apollonius of Perga (ca. 262 to 192 B.C.) was a Greek geometer and astronomer known for his writings on conics. Hypsicles (ca. 190 to 120 B.C.) was a Greek mathematician and astronomer known for authoring 'On Ascensions' and Book XIV of Euclid's Elements. The book continues Euclid's comparison of regular solids inscribed in spheres. Hipparchus of Nicaea (190 to 120 B.C.) was a Greek astronomer, geographer, and mathematician. He is considered the founder of trigonometry but is most famous for his incidental discovery of precession of the equinoxes. He is known to have compiled the first trigonometric table --- a table of values of the trigonometric chord of angles. (This chord is sometimes written as 'crd'.) Theodosius of Bithynia (ca. 160 to 100 B.C.) was a Greek astronomer and mathematician who wrote the 'Sphaerics', a book on the geometry of the sphere. Two other works by Theodosius have survived: 'On Habitations', describing the appearances of the heavens at different climes, and 'On Days and Nights', a study of the apparent motion of the Sun. We switch to A.D. here --- or as they say nowadays C.E. (Common Era) Claudius Ptolemy (83 to 161 A.D.) was a Greco-Roman writer of Alexandria, known as a mathematician, astronomer, geographer, and astrologer. He lived in the city of Alexandria in the Roman province of Egypt, wrote in Greek, and held Roman citizenship. He believed in an Earth-centered universe and provided a means of (roughly) predicting the motions of heavenly bodies based on circles as their paths --- or 'epicycles', where an epicycle is a circle whose center follows a circular path. Ptolemy presented a useful tool for astronomical calculations in his 'Handy Tables', which tabulated all the data needed to compute the positions of the Sun, Moon and planets, the rising and setting of the stars, and eclipses of the Sun and Moon. Pappus of Alexandria (ca. 290 to 350 A.D.) was one of the last great Greek mathematicians of Antiquity, known for his Synagoge or Collection (c. 340), and for Pappus's Theorem in projective geometry. His work had applications in astronomy. Theon_of_Alexandria (ca. 335 to 405 A.D.) was a Greco-Egyptian scholar and mathematician who lived in Alexandria, Egypt. He edited and arranged Euclid's Elements and wrote commentaries on works by Euclid and Ptolemy. His daughter Hypatia also won fame as a mathematician, but she was murdered by a Christian mob. Theon is said to have written a work on the astrolabe. Here we enter the Dark Ages of Europe, when many Christians suspected mathematics to be the work of the devil. For the next 1,100 years or so, mathematical works are preserved and advanced elsewhere, notably in the Middle East and India. Some of the ancient Alexandrian texts (that were not burned by the Romans ca. 48 B.C. --- or Christians ca. 392 A.D. --- or Muslims ca. 640 A.D.) were translated into Arabic, and, much later, back into Greek and Latin. Aryabhata (475 to 550? A.D.) was an Indian astronomer and mathematician. He worked on the approximation for pi, equations, and series. He found that 'the sum of cubes is the square of the sum' : 1 + 2^3 + 3^3 + ... + n^3 = ( 1 + 2 + 3 + ... + n )^2 . (It blows my mind that this is true for all positive integers n.) Also, he found that the ratio of the circumference to the diameter of a circle is 62832/20000 = 3.1416, which is accurate to five significant figures. Brahmagupta (598 to 665 A.D.) was an Indian mathematician and astronomer. He wrote out a set of rules to compute with the number zero. It took a while for people to recognize that having the number zero is quite helpful in many ways --- if only as a place holder in representations of large numbers. Al-Khwarizmi (Al-Khowarizmi, Mohammed ibn Musa) (ca. 780 to 850 A.D.) was a Persian mathematician, astronomer and geographer during the Abbasid Empire, a scholar in the House of Wisdom in Baghdad. He wrote a book 'Kitab wa al jabr wa al muqabalah' (The Book of Shifting and Balancing) which described six types of quadratic equation and solved them in a methodical manner. His works introduced the decimal positional number system to the Western world. The words 'al jabr' are the source of the English word 'algebra'. A very influential person. Albtegnius al-Battani (ca. 858 to 929 A.D.) was an Arab Muslim astronomer, astrologer, and mathematician. He introduced a number of trigonometric relations, and he authored a book of astronomical tables that was frequently quoted by many medieval astronomers, including Copernicus. One of his best-known achievements was the determination of the solar year as being 365 days, 5 hours, 46 minutes and 24 seconds. Habash al-Hasib (Ahmed ibn Abdallah al-Mervazi) (ca. 870 A.D.) was a Persian astronomer, geographer, and mathematician. He flourished in Baghdad and died in Samarra, Iraq. In 830, using the notion of "shadow" --- equivalent to our tangent in trigonometry --- he compiled a table of such shadows which seems to be the earliest of its kind. He also introduced the cotangent, and produced the first tables for it. He provided estimates of the circumference and diameter of the earth, moon, and sun --- and the min-max distances between the earth and the moon and the distance between the earth and the sun. Abul al-Wafa (940 to 998 A.D.) was a Persian mathematician and astronomer who worked in Baghdad. He made important innovations in spherical trigonometry, and his work on arithmetics for businessmen contains the first instance of using negative numbers in a medieval Islamic text. He is also credited with compiling the tables of sines and tangents at 15' intervals. He also introduced the sec and cosec functions. He established several trigonometric identities such as sin(a ± b) in their modern form, where the Ancient Greek mathematicians had expressed the equivalent identities in terms of chords. He also discovered the law of sines for spherical triangles: sin A/sin a = sin B/sin b = sin C/sin c where A, B, C are the sides and a, b, c are the opposing angles. Omar Khayyam (1048 to 1131 A.D.) was a Persian philosopher, mathematician, astronomer and poet. He also wrote treatises on mechanics, geography, mineralogy, music, and Islamic theology. He provided an algebraic method of solving certain cubic equations, while others, such as Menaechmus, provided geometric methods. Gherardo of Cremona (1114? to 1187) was an Italian-born translator of scientific books from Arabic into Latin. He worked in Toledo, Spain and obtained the Arabic books in the libraries at Toledo. Some of the books had been originally written in Greek and were unavailable in Greek or Latin in Europe at the time. Gerard of Cremona is the most important translator among the Toledo School of Translators who invigorated medieval Europe in the twelfth century by transmitting medieval Arabic and ancient Greek knowledge in astronomy, medicine and other sciences, by making the knowledge available in the Latin language. One of Gerard's most famous translations is of Ptolemy's Almagest from Arabic texts found in Toledo. Nicole Oresme (1320-1325? to 1382) was a French theologian. He wrote influential works on economics, mathematics, physics, astrology and astronomy, philosophy, and theology. He was Bishop of Lisieux, a translator, and a counselor of King Charles V of France. He is known for developing the first proof of the divergence of the harmonic series --- 1 + 1/2 + 1/3 + 1/4 + ... Several centuries later, the Bernoulli brothers did not know of his proof and came up with their own. Ulugh Begh (Mirza Mohammad Taraghay bin Shahrukh) of Samarkand (currently in Uzbekistan) (1393 to 1449) was a Timurid (Middle East) ruler as well as an astronomer, mathematician and sultan. Ulugh Beg was notable for his work in astronomy-related mathematics, such as trigonometry and spherical geometry. He built the great Ulugh Beg Observatory in Samarkand between 1424 and 1429. It was considered by scholars to have been one of the finest observatories in the Islamic world at the time and the largest in Central Asia. He wrote accurate trigonometric tables of sine and tangent values correct to at least eight decimal places. George of Trebizond (1396 to 1486) was a Greek philosopher and scholar, who migrated to Italy and became one of the pioneers of the Renaissance. His numerous works consisted of translations from Greek into Latin and original essays in Greek. He wrote a commentary on Ptolemy's Almagest. Nicolaus Cusanus (1401 to 1464) was a German philosopher, theologian, jurist, and astronomer. Most of Nicholas of Cusa's mathematical ideas can be found in his essays, 'De Docta Ignorantia' (Of Learned Ignorance), 'De Visione Dei' (On the Vision of God) and 'On Conjectures'. He also wrote on squaring the circle in his mathematical treatises. His astronomical views evince complete independence of traditional doctrines. The earth is a star like other stars, is not the centre of the universe, is not at rest, nor are its poles fixed. The celestial bodies are not strictly spherical, nor are their orbits circular. Had Copernicus been aware of these assertions, he would probably have been encouraged by them to publish his own monumental work. Leone Battista Alberti (1404 to 1472 A.D.), an Italian architect and artist, raised the ideas of vanishing point (a term coined later) and perspective in his treatise, 'Della Pittura' ('On Painting'). It is claimed that that treatise contained the first scientific study of perspective. He worked with the astronomer, astrologer, and cartographer Paolo Toscanelli (1397-1482). Georg von Peurbach (also Purbach, Peurbach, Purbachius, his real surname is unknown) (1423 to 1461) was an Austrian astronomer, mathematician, and instrument maker. Peurbach has been called the father of mathematical and observational astronomy in the West. He began to work up Ptolemy's Almagest and Alhazen's 'On the Configuration of the World'. He replaced Ptolemy's chords with the sines from Arabic mathematics, and calculated tables of sines for every minute of arc for a radius of 600,000 units. This was the first transition from the duodecimal to the decimal system. Perubach taught in Vienna. His most famous pupil was Johann Müller of Königsberg, later known as Regiomontanus. Upon the death of Peurbach, Regiomontanus finished several of the works started by Purbach. Johann Muller of Konigsberg (later known as Regiomontanus) (1436 to 1476) was a German mathematician, astronomer, astrologer, translator, instrument maker and Catholic bishop. His work on arithmetic and algebra, 'Algorithmus Demonstratus', was among the first containing symbolic algebra. In 'Epytoma in almagesti Ptolemei', he critiqued the translation of Almagest by George of Trebizond, pointing out inaccuracies. Later Nicolaus Copernicus would refer to this book as an influence on his own work. He founded the world's first scientific printing press and in 1472 he published the first printed astronomical textbook, the 'Theoricae novae Planetarum' of his teacher Georg von Peurbach. A prolific author, Regiomontanus was internationally famous in his lifetime. Despite having completed only a quarter of what he had intended to write, he left a substantial body of work. Christopher_Columbus (1451 to 1506) was an Italian explorer, navigator, and colonizer, born in the Republic of Genoa, in today's northwestern Italy. His dates are placed here mainly to compare with the dates of physicists listed here, thus giving some sense of the state of mathematics and astronomy at the time of Columbus's voyages. Columbus learned Latin, as well as Portuguese and Castilian, and read widely about astronomy, geography, and history, including the works of Claudius Ptolemy. Nicolas Copernicus (1473 to 1543) was a Polish astronomer and mathematician who provided much evidence that a sun-centered universe explained many phenomena that were not explained well by an earth-centered universe. His theory was made public in his book 'De Revolutionibus Orbim Coelestium' ('On the Revolutions of the Heavenly Orbs'), which is often credited as being the beginning of the so-called 'Scientific Revolution'. Some claim that the book was not published until he was near death because he knew it could be dangerously controversial --- as Galileo was to find out. (It seems it took Newton to raise mathematics out of the Dark Ages.) Ferdinand Magellan (ca. 1480 to 1521) was a Portuguese explorer. Magellan's expedition of 1519-1522 became the first expedition to sail from the Atlantic Ocean into the Pacific Ocean, and the first to cross the Pacific. His expedition completed the first circumnavigation of the Earth, although Magellan himself did not complete the entire voyage, being killed during the Battle of Mactan in the Philippines. His dates are placed here mainly to compare with the dates of physicists listed here, thus giving some sense of the state of mathematics and astronomy at the time of Magellan's voyages. Magellan no doubt used astronomical (navigation) information from Ptolomy or Copernicus or Arabian astronomers-and-cartographers, either directly from translations of their works or indirectly via 'commentaries' or re-working of those works. Francesco Maurolico (1494 to 1575) was a Greek mathematician and astronomer of Sicily. The proof by 'mathematical induction' had been used implicitly for centuries by Greek, Indian, and Arab mathematicians, but Maurolico first stated it formally. Basically: If S is a statement about integers: Show that S is true for n=1. Show that if S is true for n=k, then it is also true for n=k+1. Then one can declare that S is true for all positive integers. Throughout his lifetime, Maurolico made contributions to the fields of geometry, optics, conics, mechanics, music, and astronomy. He edited the works of classical authors including Archimedes, Apollonius, Autolycus, Theodosius and Serenus. He also composed his own unique treatises on mathematics. Gerolamo Cardano (1501 to 1576) was an Italian Renaissance mathematician, physician, astrologer and gambler. He wrote more than 200 works on medicine, mathematics, physics, philosophy, religion, and music. His gambling led him to formulate elementary rules in probability, making him one of the founders of the field. He was a teacher of Lodovico Ferrari (see below). Pedro Nunes (1502 to 1578) was a Portuguese mathematician, cosmographer, and professor. Nunes, considered to be one of the greatest mathematicians of his time, is best known for his contributions in the technical field of navigation, which was crucial to the Portuguese period of discoveries. He was the first to understand why a ship maintaining a steady course would not travel along a great circle, the shortest path between two points on Earth, but would instead follow a spiral course, called a loxodrome. The later invention of logarithms allowed Leibniz to establish algebraic equations for the loxodrome. In his 'Treaty defending the sea chart', Nunes argued that a nautical chart should have its parallels and meridians shown as straight lines. Yet he was unsure how to solve the problems that this caused: a situation that lasted until Mercator developed the projection bearing his name. The Mercator Projection is the system which is still used. Nunes also solved the problem of finding the day with the shortest twilight duration, for any given position, and its duration. This problem per se is not greatly important, yet it shows the geometric genius of Nunes as it was a problem which was independently tackled by Johann and Jakob Bernoulli more than a century later with less success. They could find a solution to the problem of the shortest day, but failed to determine its duration, possibly because they got lost in the details of differential calculus which, at that time, had only recently been developed. The achievement also shows that Nunes was a pioneer in solving maxima and minima problems, which became a common requirement only in the next century using differential calculus. Gemma Frisius (1508 to 1555) was a physician, mathematician, cartographer, philosopher, and (astronomical) instrument maker. He created important globes, improved the mathematical instruments of his day and applied mathematics in new ways to surveying and navigation. In 1533, he described for the first time the method of triangulation still used today in surveying. Twenty years later, he was the first to describe how an accurate clock could be used to determine longitude. His students included Gerardus Mercator (who became his collaborator), Johannes Stadius, John Dee, Andreas Vesalius and Rembert Dodoens. Gerhardus Mercator (1512 to 1594) was a 'Belgian/German' cartographer, cosmographer, philosopher and mathematician. He is best known for his work in cartography, in particular the world map of 1569 based on a new projection which represented sailing courses of constant bearing as straight lines. He was the first to use the term 'Atlas' for a collection of maps. Georg Joachim Rhaeticus (1514 to 1576) was a mathematician, cartographer, navigational-instrument maker, astronomer, medical practitioner, and teacher from the Austrian area. He is perhaps best known for his trigonometric tables and as Nicolaus Copernicus's sole pupil. He facilitated the publication of Copernicus's 'De revolutionibus orbium coelestium' ('On the Revolutions of the Heavenly Spheres'). Rheticus produced the first publication of six-function trigonometric tables (although the word trigonometry was not yet coined). A student, Valentin Otto, oversaw the hand computation of approximately 100,000 ratios to at least ten decimal places. When completed in 1596, the volume, 'Opus palatinum de triangulus', filled nearly 1,500 pages. Its tables were accurate enough to be used in astronomical computation into the early twentieth century. Cornelius Gemma (1535 to 1577?) was a physician, astronomer and astrologer, and the oldest son of cartographer and instrument-maker Gemma Frisius (see above). As an astronomer, Gemma is significant for his observations of a lunar eclipse in 1569 and of the 1572 supernova appearing in Cassiopeia. His predictions for 1561 provided detailed information on every lunar phase, and most planetary aspects and phases of fixed stars in relation to the sun, with a thoroughness that surpassed the predictions of his contemporaries. In his medical writings, in 1552, he published the first illustration of a human tapeworm. He remained committed to astrologic medicine, however, and believed that astral conjunctions generated disease. Gemma died around 1578 in an epidemic of the plague, to which a third of the population at Leuven also succumbed. He was only in his mid-forties. Clavius (1538 to 1612) was a German Jesuit mathematician and astronomer who modified the proposal of the modern Gregorian calendar after the death of its primary author, Luigi Lilio. His math works included a 'Commentary on Euclid' (1574), 'Geometrica Practica' (1604), and 'Algebra' (1608). His mathematical works (in 5 volumes) are available online. Francois Viete (Latin: Franciscus Vieta) (1540 to 1603) was a French mathematician whose work in algebraic equations was an important step towards modern algebra, due to its innovative use of letters as parameters in equations. He is also known for discovering the first infinite product in the history of mathematics --- a product which was is equal to pi. Between 1564 and 1568, Vieta prepared for his student, Catherine de Parthenay, some textbooks of astronomy and trigonometry. Tycho Brahe (1546 to 1601) was a Danish nobleman known for his accurate and comprehensive astronomical and planetary observations. Tycho was well known in his lifetime as an astronomer and alchemist. He refuted the Aristotelian belief in an unchanging celestial realm. His precise measurements indicated that "new stars," (stellae novae, now known as supernovae) lacked the parallax expected in sub-lunar phenomena, and were therefore not "atmospheric" tailless comets as previously believed, but were above the atmosphere and moon. Using similar measurements he showed that comets were also not atmospheric phenomena, as previously thought. But he did not believe the earth could orbit the sun, because he could not detect any parallax effect in observing the stars. He was the last of the major naked eye astronomers, working without telescopes for his observations. Tycho worked to combine the geo-centric Copernican system with the helio-centric Ptolemaic system into his own model of the universe. In his "geo-helio-centric" system, the sun, moon, and stars circle a central Earth, while the five planets orbit the Sun. Although Tycho's planetary model was discredited within the next 100 to 150 years, his astronomical observations were an essential contribution to the scientific revolution. John Napier (1550 to 1617) was a Scottish landowner, mathematician, physicist, and astronomer. He used logarithms (exponents) to reduce multiplication and division to addition and subtraction. The process of multiplying two numbers: Look up their logarithms in a logarithm table. Add the two logarithms. Look up the anti-logarithm of that logarithm in an anti-logarithm table. Jobst Burgi (1552 to 1632) was a Swiss clockmaker, a maker of astronomical instruments, and a mathematician. Among his major inventions were the cross-beat escapement, and the remontoire, two mechanisms which improved the accuracy of mechanical clocks of the time by orders of magnitude. This allowed clocks to be used, for the first time, as scientific instruments, with enough accuracy to time the passing of stars (and other heavenly bodies) in the crosshairs of telescopes to start accurately charting stellar positions. Besides clocks, he also made mechanized celestial globes, and he made sextants for Kepler (see below). He invented logarithms independently of John Napier, since his method is distinct from Napier's. There is evidence that Bürgi arrived at his invention as early as 1588, six years before Napier began work on the same idea. By delaying the publication of his work to 1620, Bürgi lost his claim for priority in historic discovery. Bürgi was also a major contributor to prosthaphaeresis, a technique for computing products quickly using trigonometric identities, which predated logarithms. Edward Wright (1558 to 1615) was an English mathematician and cartographer noted for his book 'Certaine Errors in Navigation' (1599), which for the first time explained the mathematical basis of the Mercator projection, and set out a reference table giving the linear scale multiplication factor as a function of latitude, calculated for each minute of arc up to a latitude of 75°. This was in fact a table of values of the integral of the secant function, and was the essential step needed to make practical both the making and the navigational use of Mercator charts. (Mercator had not explained his method.) A skilled designer of mathematical instruments, Wright made models of an astrolabe and a pantograph, and a type of armillary sphere. In the 1610 edition of 'Certaine Errors', he described inventions such as the "sea-ring" that enabled mariners to determine the magnetic variation of the compass, the sun's altitude and the time of day in any place if the latitude was known; and a device for finding latitude when one was not on the meridian using the height of the pole star. Wright translated John Napier's pioneering 1614 work which introduced the idea of logarithms from Latin into English. Wright's work influenced, among other persons, Dutch astronomer and mathematician Willebrord Snellius; Adriaan Metius, the geometer and astronomer from Holland; and the English mathematician Richard Norwood, who calculated the length of a degree on a great circle of the earth (367,196 feet ; 111,921 m) using a method proposed by Wright. John Collins, in "Navigation by the Mariners Plain Scale New Plain'd" (1659), stated that Mercator's chart ought "more properly to be called Wright's chart". Thomas Harriot (1560 to 1621) was an English astronomer, mathematician, ethnographer, and translator. After his graduation from Oxford in 1580, Harriot was first hired by Sir Walter Raleigh as a mathematics tutor. He used his knowledge of astronomy/astrology to provide navigational expertise, help design Raleigh's ships, and serve as his accountant. Prior to his expedition to the Americas with Raleigh (to Roanoke Island, 1585), Harriot wrote a treatise on navigation. In addition, he made efforts to communicate with Manteo and Wanchese, two Native Americans who had been brought to England. Harriot deciphered a phonetic alphabet to transcribe their Carolina Algonquian language. As a scientific adviser during the voyage, Harriot was asked by Raleigh to find the most efficient way to stack cannon balls on the deck of the ship. His ensuing theory about the close-packing of spheres shows a striking resemblance to atomism and modern atomic theory, which he was later accused of believing. His correspondence about optics with Johannes Kepler, in which he described some of his ideas on sphere-stacking, later influenced Kepler's conjecture on 'sphere-packing'. Halley's Comet in 1607 turned Harriot's attention towards astronomy. In early 1609 he bought a "Dutch trunke" (telescope), invented in 1608, and his observations were amongst the first uses of a telescope for astronomy. Harriot is now credited as the first astronomer to draw an astronomical object after viewing it through a telescope. He drew a map of the Moon on July 26, 1609, preceding Galileo by several months. The observatory in the campus of the College of William and Mary (Williamsburg, Virginia) is named in Harriot's honour. Harriot apparently died of a cancer that started on his lip. Harriot's accomplishments remain relatively obscure because he did not publish any of his results and also because many of his manuscripts have been lost. Those that survive are sheltered in the British Museum and in the archives of the Percy family at Petworth House (Sussex) and Alnwick Castle (Northumberland). Thomas Fincke (1561 to 1646) was a Danish mathematician and physicist, and a professor at the University of Copenhagen for more than 60 years. His lasting achievement is found in his book 'Geometria rotundi' (1583), in which he introduced the modern names of the trigonometric functions tangent and secant. Henry Briggs (1561 to 1630) was an English mathematician and astronomer notable for changing the original logarithms invented by John Napier into common (base 10) logarithms. He published Napier's logarithms as simplified tables and helped them gain acceptance among the scientific and academic communities. Bartholomaeus Pitiscus (1561 to 1613) was a 16th-century German trigonometrist, astronomer and theologian who first coined the word Trigonometry. Pitiscus is sometimes credited with inventing the decimal point, the symbol separating integers from decimal fractions, which appears in his trigonometrical tables and was subsequently accepted by John Napier in his logarithmic papers (1614 and 1619). Pitiscus edited 'Thesaurus mathematicus' (1613) in which he improved the trigonometric tables of Georg Joachim Rheticus. Johan Philip Lansberge (1561 to 1632) was a Dutch Calvinist minister, astronomer and mathematician. He is best known as the author of a set of astronomical tables for predicting planetary positions. Kepler and Galileo, who lived in the same period, were very interested in the work of Lansbergen. Based on his tables, they could predict the movements of the planets more accurately. Galileo Galilei (1564 to 1642) was an Italian physicist, mathematician, and astronomer. He provided rules for how a body falls under gravity. For example: If a body is dropped from rest, the distance it falls varies with the square of the time. Other discoveries by Galileo : The first thermometer. Used the telescope to discover the moons of Jupiter. Used the telescope to show that the Moon has mountains and valleys. A pendulum swings to and fro in the same time, regardless of the angle through which it swings. (This is about as counter-intuitive as the the fact that a heavy body and a light body fall at the same speed. Aristotle believed the latter --- but, unlike Galileo, Aristotle was loathe to put his 'logic' to experimental test.) Also Galileo was a leading supporter of Copernicus's Sun-centered system --- which led to his spending his last years under house arrest due to the influence of the Catholic Church. Johannes Kepler (1571 to 1630) was a German mathematician, astronomer and astrologer. Using copious data on planetary movements, he provided three laws about the motion of planets. The first and most revolutionary: Planets travel in ellipses rather than circles. Isaac Newton was later able to deduce the 3 Kepler laws based on his laws of motion and of gravity. Also, Kepler showed that the square, triangle and hexagon are the only regular polygons that can be used to cover a plane without leaving any gaps. This is known as a 'tesselation' or 'tiling' of the plane.

Ellipses are approximations, but good ones for our solar system.
Newton pointed out that other planets influence
the orbits of any one planet. But, fortunately,
the Sun's mass is so great, it dominates ---
gravitationally speaking.

Not only did Kepler notice that planets move faster
when closer to the Sun, but he quantified the motion
and made the observation about equal areas.
(Perhaps he concluded that equal arc-distances did not work.)
What a guy. Quite a genius of his time.
Kepler's contributions are not given enough weight.
He (and Galileo and others) really gave Newton 'a leg up'.

William Oughtred (1574 to 1660) was an English mathematician. After John Napier invented logarithms, and Edmund Gunter created the logarithmic scales (lines, or rules) upon which slide rules are based, it was Oughtred who first used two such scales sliding by one another to perform direct multiplication and division; and he is credited as the inventor of the slide rule in 1622. Gunter required the use of a pair of dividers, to lay off distances on his rule; Oughtred made the step of sliding two rules past each other to achieve the same ends. Oughtred also introduced the "×" symbol for multiplication as well as the abbreviations "sin" and "cos" for the sine and cosine functions. Oughtred published 'Clavis Mathematicae' (The Key to Mathematics) in 1631. It became a classic, reprinted in several editions, and used by Wallis and Isaac Newton (see below) amongst others. It was not ambitious in scope, but aimed to represent current knowledge of algebra concisely. It argued for a less verbose style of mathematics, with a greater dependence on symbols. Mathurin Jousse (ca. 1575? to 1645) was a French inventor and a technician in iron works. He was also said to be an "engineer and architect of the town of de La Flèche". It is said that he carried out several repair/construction jobs of, large and small, for the town and the Jesuit college there, such as repair of the large clock on the Saint-Thomas bell tower. Jousse was curious about science and techniques. He possessed scientific instruments, some he made himself, and a rich library in which there were many books of arithmetic, geometry and astronomy. His treatise "Secret d'architecture", entirely devoted to stereotomy, appeared in La Flèche in 1642. It is said that he knew Francois Derand (see below), a student at the Jesuit college in La Fleche who also wrote a text on stereotomy. Johann Faulhaber (1580 to 1635) was a German mathematician. Born in Ulm, Faulhaber trained as a weaver and later took the role of a surveyor of the city of Ulm. He collaborated with Johannes Kepler and Ludolph van Ceulen (see above). Besides his work on the fortifications of cities (notably Basel and Frankfurt), Faulhaber built water wheels in his home town and geometrical instruments for the military. Faulhaber supervised the first publication of Henry Briggs's logarithms in Germany. Faulhaber's major math contribution involved calculating the sums of powers of integers. Jacob Bernoulli makes references to Faulhaber in his 'Ars Conjectandi'. Faulhaber made a major impression on Descartes and influenced his thinking. In 1631, Faulhaber published 'Academia Algebra'. Edmund Gunter (1580 to 1626) was an English mathematician, of Welsh descent. In 1619, Gunter was appointed professor of astronomy in Gresham College, London. This post he held till his death. In 1620, he published his 'Canon triangulorum'. With Gunter's name are associated several useful inventions, descriptions of which are given in his treatises on the Sector, Cross-staff, Bow, Quadrant and other instruments. In 1624, Gunter published a collection of his mathematical works. It was entitled 'The description and use of sector, the cross-staffe, and other instruments for such as are studious of mathematical practise'. It was a manual not for cloistered university fellows but for sailors and surveyors in real world. It was written, and published, in English not Latin. Willebrord Snellius van Roijen 'Snell' (1581 to 1626) was a Dutch astronomer and mathematician. In the west, especially the English speaking countries, his name has been attached to the law of refraction of light for several centuries, but it is now known that this law was first discovered by Ibn Sahl in 984. The same law was also investigated by Ptolemy and in the Middle Ages by Witelo, but due to lack of adequate mathematical instruments (trigonometric functions) their results were saved as tables, not functions. In 1615, he planned and carried into practice a new method of finding the radius of the earth, by determining the distance of one point on its surface from the parallel of latitude of another, by means of triangulation. His work 'Eratosthenes Batavus' ("The Dutch Eratosthenes"), published in 1617, describes the method. Snellius also produced a new method for calculating pi --- the first such improvement since ancient times. Gregoire de Saint-Vincent (1584 to 1667) was a Flemish Jesuit and mathematician. Saint-Vincent discovered that the area under a rectangular hyperbola ( i.e. a curve given by xy = k ) is the same over [a,b] as over [c,d] when a/b = c/d. This discovery was fundamental for the development of the theory of logarithms and an eventual recognition of the natural logarithm (whose series representation was discovered by Nicholas Mercator (not to be confused with Gerardus Mercator the cartographer), but was only later recognized as a log of base e). The stated property allows one to define a function A(x) which is the area under said curve from 1 to x, which has the property that A(xy) = A(x)+A(y). Since this functional property characterizes logarithms, it has become mathematical fashion to call such a function A(x) a logarithm. In particular when we choose the rectangular hyperbola xy = 1, one recovers the natural logarithm. To a large extent, recognition of de Saint-Vincent's achievement in quadrature of the hyperbola is due to his student and co-worker Alphonse Antonio de Sarasa, with Marin Mersenne (see below) acting as catalyst. It was in attempting to 'square the circle' that Saint-Vincent made these discoveries. In the Complete Works of Huygens, there is a section on the 'cyclometrie' of Gregoire de Saint-Vincent (page 374). Isaac Beeckman (1588 to 1637) was a Dutch philosopher and scientist. Rejecting Aristotle, Beeckman developed the concept that matter is composed of atoms. In 1618, he became a teacher and friend of René Descartes. He convinced Descartes to devote his studies to a mathematical approach to nature. When Descartes returned to the Dutch Republic in the autumn of 1628, Beeckman also introduced him to many of Galileo's ideas. In his time, Beeckman was considered to be one of the most educated men in Europe. For example, he had deeply impressed Mersenne, despite their opposing religious views. Marin Mersenne (1588 to 1648), a French priest, conjectured that all numbers of the form 2^n - 1, where n is prime, are prime. It turns out that 2^11 - 1 is 23 x 89, and hence not prime. If it were true, we would have a simple formula for finding infinitely many primes. For n = 2,3,5,7, the Mersenne numbers are 3, 7, 31, 127 --- all prime. Note that Euclid (ca. 325-265 B.C.) showed that there are infinitely many primes, but he did not find a rule for generating a succession of them. In later life, Mersenne gave up speculative thought and turned to scientific research, especially in mathematics, physics and astronomy. He also performed extensive experiments to determine the acceleration of falling objects by comparing them with the swing of pendulums. Thomas Hobbes (1588 to 1679) was an English philosopher, best known today for his work on political philosophy. His mathematics contributions are not very significant, but he got into a long dispute with John Wallis, who was second only to Isaac Newton as the leading English mathematician of their age. In addition to political philosophy, Hobbes also contributed to a diverse array of other fields, including the physics of gases. Richard Norwood (1590 to 1665) was an English mathematician, diver, and surveyor. In 1616, he was sent to survey the islands of Bermuda (also known as the Somers Isles). He was (around 1630 to 1640) a teacher of mathematics in London. Between 1633 and 1635, he personally measured, partly by chain and partly by pacing, the distance between London and York, making corrections for all the windings of the way, as well as for the ascents and descents. He also, from observations of the sun's altitude, computed the difference of latitude of the two places, and so calculated the length of a degree of the meridian. His result was some 600 yards too great; but it was the nearest approximation that had then been made in England. (See Edward Wright above.) Isaac Newton noted Norwood's work in his 'Principia Mathematica'. Norwood is credited with founding Bermuda's oldest school, Warwick Academy, in 1662. He died at Bermuda in 1675, aged about eighty-five, and was buried there. He has been called "Bermuda's outstanding genius of the seventeenth century" (in spite of some alleged collusion with the governor during the 1616 survey) --- probably by Bermudans eager for a noted Bermudan. Girard Desargues (1591 to 1661) was a French mathematician and engineer, and is considered one of the founders of projective geometry. Desargues' theorem and the Desargues graph are named in his honor. His research on perspective and geometrical projections can be seen as a culmination of centuries of scientific inquiry across the classical epoch in optics that stretched from al-Hasan Ibn al-Haytham (Alhazen) to Johannes Kepler, and going beyond a mere synthesis of these traditions with Renaissance perspective theories and practices. One notable work, often cited by others in mathematics, is "Rough draft for an essay on the results of taking plane sections of a cone" (1639). Pierre Gassendi (1592 to 1655) was a French philosopher, priest, scientist, astronomer, and mathematician. In 1645, he accepted the chair of mathematics in the Collège Royal in Paris, and lectured for several years. In 1648, ill-health compelled him to give up his lectures at the Collège Royal. He had published criticisms of Decartes' views, but around this time he became reconciled to Descartes, after years of coldness. In his dispute with Descartes, Gassendi apparently held that the evidence of the senses remains the only convincing evidence; yet he maintains, as is natural from his mathematical training, that the evidence of reason is absolutely satisfactory. Wilhelm Schickard (1592 to 1635), a professor of astronomy, designed a machine that could perform the four basic arithmetic operations - addition, subtraction, multiplication, and division. Unfortunately, before it was completed, it was destroyed in a fire, but sufficient details survived for the machine to be successfully reconstructed in the 1950's. Albert Girard (1595 to 1632) was a French-born mathematician. He gave the inductive definition for the Fibonacci numbers. He was the first to use the abbreviations 'sin', 'cos' and 'tan' for the trigonometric functions in a treatise. Girard was the first to state, in 1632, that each prime of the form 4n+1 was the sum of two squares. Girard also showed how the area of a spherical triangle depends on its interior angles. The result is called Girard's theorem. Jacobus_Golius (born Jacob van Gool) (1596 to 1667) was a Dutch mathematician and a student of Arabic and Eastern languages. Golius taught mathematics to the French philosopher René Descartes, and later corresponded with him. It is therefore highly probable that he was able to read to him parts of the mathematical Arabic texts he had started to collect, among others on the Conics. Golius edited, translated and annotated the astronomical treatise of the 9th century Arabic astronomer Al-Farghani. Rene Descartes (1596 to 1650), a French 'natural philosopher', physiologist, and mathematician, was the first to systematically develop the idea of analytic (or Cartesian, or coordinate) geometry --- think 'graph paper'. To some extent, modern philosophy began with his "Cogito ergo sum" --- "I think, therefore I am." Newton's presentation of the 'law of gravitational attraction' eventually displaced Descartes' theory of vortices to explain the motion of planets. Bonaventura Cavalieri (1598 to 1647) was an Italian mathematician. He is known for his work on the problems of optics and motion, work on the precursors of infinitesimal calculus, and the introduction of logarithms to Italy. 'Cavalieri's principle' in geometry partially anticipated integral calculus. 'Cavalieri's principle' states that the volumes of two objects are equal if the areas of their corresponding cross-sections are in all cases equal. The same principle had been previously discovered by Zu Gengzhi (480-525) of China. It was a significant step on the way to modern infinitesimal calculus. Florimond de Beaune (1601 to 1652) was a French jurist and mathematician, and an early follower of René Descartes. His 'Tractatus de limitibus aequationum' was reprinted in England in 1807. In it, he finds upper and lower bounds for the solutions to quadratic equations and cubic equations, as simple functions of the coefficients of these equations. His 'Doctrine de l'angle solide' and 'Inventaire de sa bibliothèque' were also reprinted, in Paris in 1975. Another of his writings was 'Notae breves', the introduction to a 1649 edition of Descartes' 'La Géométrie'. Pierre de Fermat (1601 to 1665) conjectured that 2^2^n + 1, where n is any positive integer, is prime. It turns out that for n=5, the result can be factored into 641 x 7,600,417. For n = 0,1,2,3,4, the Fermat numbers are 3, 5, 17, 257, and 65,537 --- all prime. Up to 2008, these are the only Fermat numbers that have been found to be prime. Fermat traded letters with Pascal on a gambling problem --- how stakes should be divided in an interrupted game --- a certain kind heads-tails game. Fermat's solution, which was refined by Pascal, was to look to the future rather than the past. Instead of considering the points that had been played, they looked at the points that would be played if the game were allowed to continue. The theory of probability is said to have begun with this gambling problem. Euclid pointed out that, for the path of light reflecting from a mirror, the angle of incidence equals the angle of reflection. Hero of Alexandria later showed that this path gave the shortest length and the least time. Fermat refined and generalized this to "light travels between two given points along the path of shortest time" now known as the principle of least time. For this, Fermat is recognized as a key figure in the historical development of the fundamental principle of least action in physics. Pierre de Carcavy (1600 or 1603? to 1684) was French and a member of the Académie Royal des Sciences, 1666-1684. He had many friends, including Huygens, Fermat, and Pascal, and carried on an extensive correspondence. He was instrumental in passing information among these 'natural philosophers'. Antoine de Laloubère (Antonius Lalovera) (1600 - 1664) was a French mathematician who gained the respect of Wallis, who had a strong influence on Newton (see below). Jacques de Billy (1602 to 1679) was a French Jesuit mathematician. From 1629 to 1668, he taught mathematics at several Jesuit colleges. Billy maintained a correspondence with the mathematician Pierre de Fermat. A couple of Billy's pupils were Jacques Ozanam and Claude Gaspard Bachet de Méziriac. Billy produced a number of results in number theory which have been named after him. Billy's mathematical works include 'Diophantus Redivivus'. In the field of astronomy, he published several astronomical tables. Billy was one of the first scientists to reject the role of astrology in science. He also rejected old notions about the malevolent influence of comets. Gilles Persone de Roberval (1602 to 1675), a French mathematician, was born at Roberval, Oise, near Beauvais, France. His name was originally Gilles Personne or Gilles Personier, that of Roberval, by which he is known, being taken from the place of his birth. He was appointed to the chair of philosophy at Gervais College in 1631, and two years later to the chair of mathematics at the Royal College of France. A condition of tenure attached to this chair was that the holder should propose mathematical questions for solution, and should resign in favour of any person who solved them better than himself; but, notwithstanding this, Roberval was able to keep the chair about 40 years, till his death. Roberval was one of those mathematicians who, just before the invention of the differential and integral calculus, occupied their attention with problems which are only soluble, or can be most easily solved, by some method involving limits or infinitesimals, which would today be solved by calculus. He worked on the quadrature of surfaces and the cubature of solids, which he accomplished, in some of the simpler cases, by an original method which he called the "Method of Indivisibles"; but he lost much of the credit of the discovery as he kept his method for his own use, while Bonaventura Cavalieri published a similar method which he independently invented. Another of Roberval's discoveries was a very general method of drawing tangents, by considering a curve as described by a moving point whose motion is the resultant of several simpler motions. He also discovered a method of deriving one curve from another, by means of which finite areas can be obtained equal to the areas between certain curves and their asymptotes. To these curves, which were also applied to effect some quadratures, Evangelista Torricelli gave the name "Robervallian lines." He invented a special kind of balance, the Roberval Balance. A Roberval balance with balanced masses. Note that the masses don't need to be centered on the plates. Martin (Maarten) van den Hove --- Latinized as Martinus Hortensius (Ortensius) --- (1605 to 1639) was a Dutch astronomer and mathematician. Van den Hove developed a method for measuring the diameters of planets based on the measured visual angle that his telescope revealed. His was probably the first independent set of measurements of the apparent sizes of the planets and fixed stars since the work of Hipparchus in his 'On Sizes and Distances' some seventeen centuries earlier. Bernard Frenicle de Bessy (1605 to 1675) was a French (amateur) mathematician, who wrote numerous mathematical papers, mainly in number theory and combinatorics. He is best remembered for 'Des quarrez ou tables magiques', a treatise on magic squares published posthumously in 1693, in which he described all 880 essentially different normal magic squares of order 4. The Frénicle standard form, a standard representation of magic squares, is named after him. He solved many problems created by Fermat and also discovered the cube property of the number 1729, later referred to as a taxicab number. He corresponded with Descartes, Huygens, Mersenne, and Fermat, who was his personal friend. Honore Fabri (1606? to 1688) was a French Jesuit theologian, mathematician, and physicist. Leibniz placed him with Galileo, Torricelli, Steno and Borelli for his work on elasticity and the theory of vibrations, and alone with Galileo for his efforts to 'rationalise experimental kinematics'. Vincentio Reinieri (1606 to 1647) was an Italian mathematician and astronomer. He was a friend and disciple of Galileo Galilei. Before his death, Galileo decided to place all of the papers containing his observations and calculations in the hands of Reinieri. Reinieri was to finish and revise them. Evangelista Torricelli (1608 to 1647) was an Italian physicist and mathematician, best known for his invention of the barometer. After Galileo's death on 8 January 1642, Grand Duke Ferdinando II de' Medici asked him to succeed Galileo as the grand-ducal mathematician and professor of mathematics at the University of Pisa. In this role, he solved some of the great mathematical problems of the day, such as finding a cycloid's area and center of gravity. He also designed and built a number of telescopes and simple microscopes. Several large lenses, engraved with his name, are still preserved in Florence. Torricelli also discovered Torricelli's Law, regarding the speed of a fluid flowing out of an opening, which was later shown to be a particular case of Bernoulli's principle. Torricelli gave the first scientific description of the cause of wind: "... winds are produced by differences of air temperature, and hence density, between two regions of the earth." Torricelli is also famous for the discovery of the Torricelli's trumpet (also - perhaps more often - known as Gabriel's Horn) whose surface area is infinite, but whose volume is finite. This was seen as an "incredible" paradox by many at the time, including Torricelli himself, and prompted a fierce controversy about the nature of infinity.

Cycloid genererated by a fixed point on a rolling circle.

Giovanni Alfonso Borelli (1608 to 1679) was an Italian physiologist, physicist, and mathematician. He contributed to the modern principle of scientific investigation by continuing Galileo's custom of testing hypotheses against observation. Trained in mathematics, Borelli also made extensive studies of Jupiter's moons, the mechanics of animal locomotion and, in microscopy, of the constituents of blood. He also used microscopy to investigate the stomatal movement of plants, and undertook studies in medicine and geology. During his career, he enjoyed the patronage of ex-Queen Christina of Sweden, who had also been exiled to Rome for converting to Catholicism. Borelli's major scientific achievements are focused around his investigation into biomechanics. The American Society of Biomechanics uses the Borelli Award as its highest honour for research in the area. Borelli is also considered to be the first man to consider a self-contained underwater breathing apparatus along with his early submarine design. Borelli also had interests in physics, specifically the orbits of the planets. Borelli's measurements of the orbits of satellites of Jupiter are mentioned in Volume 3 of Newton's Principia. Jan Mikolaj Smoguleski (1610 to 1656) was a Polish nobleman, politician, scholar, and Jesuit missionary credited with introducing logarithms to China. He also taught astronomy and mathematics in China and was much respected by Chinese scholars. His fame as a scholar and teacher spread, and in 1653 he was invited by the Shunzhi Emperor to his court in Beijing. After further travels in Asia, he died in China. John Pell (1611 to 1685) was an English mathematician. Diophantine equations was a favorite subject with Pell. He lectured on them when he taught for a while in Amsterdam. He is now best remembered, erroneously, for the indeterminate equation x^2 - n * y^2 = 0, where n is a non-square integer, which is known as Pell's equation. Pell's equation. Its solutions may be used to accurately approximate the square root of n by rational numbers of the form x/y. One of his works was titled 'Astronomical History of Observations of Heavenly Motions and Appearances' (1634). Claude Clersellier (1614 to 1684) was a French government employee (with a large dowry) who admired Descartes and published many of Descartes' papers, and translated many into French. Phillipe de la Hire (1614 to 1718) was a French mathematician and astronomer. La Hire wrote on graphical methods, 1673; on conic sections , 1685; a treatise on epicycloids , 1694; one on roulettes , 1702; and, lastly, another on conchoids , 1708. His works on conic sections and epicycloids were founded on the teaching of Desargues (see above), of whom he was a pupil. Not surprisingly then, la Hire did work on mathematics of perspective geometry.

Generation of a 'roulette' curve.

Generation of a (repeating, periodic) 'epicycloid' curve.

Frans van Schooten Jr. (1615 to 1660) was a Dutch mathematician who is most known for popularizing the analytic geometry of René Descartes. In 1646, he published a collection of Vieta's writings. Van Schooten's father was a professor of mathematics at Leiden, having Christiaan Huygens, Johann van Waveren Hudde, and René de Sluze as students. In 1646, he inherited his father's position and one of his pupils, Huygens. Van Schooten's 1649 Latin translation of and commentary on Descartes' 'Géométrie' was valuable in that it made the work comprensible to the broader mathematical community, and thus was responsible for the spread of analytic geometry to the world. A later 2-volume edition (of 1659 and 1661) was the edition that Gottfried Leibniz and Isaac Newton knew. Van Schooten was one of the first to suggest, in exercises published in 1657, that the analytic geometry ideas be extended to three-dimensional space. Van Schooten's efforts also made Leiden the centre of the mathematical community for a short period in the middle of the seventeenth century. John Wallis (1616 to 1703) was an English mathematician who is given partial credit for the development of infinitesimal calculus. His works were read by Newton, and they had great influence on Newton. Between 1643 and 1689, Wallis served as chief cryptographer for Parliament and, later, the royal court. He was appointed in 1649 to be the Savilian Chair of Geometry at Oxford University, where he lived until his death in 1703. Wallis made significant contributions to algebra, trigonometry, geometry, and the analysis of infinite series. In 1655, Wallis published a treatise on conic sections in which they were defined analytically. This was the earliest book in which these curves are considered and defined as curves of the second degree. It helped to remove some of the perceived difficulty and obscurity of René Descartes' work on analytic geometry. Wallis had an interesting view of the 'infinitesimal' lines that make up a plane: In his 'Treatise on the Conic Sections', Wallis wrote, "I suppose any plane (following the 'Geometry of Indivisibles' of Cavalieri) to be made up of an infinite number of parallel lines, or as I would prefer, of an infinite number of parallelograms of the same altitude; (let the altitude of each one of these be an infinitely small part of the whole altitude, ... and the altitude of all to make up the altitude of the figure." This view was probably useful in his work on quadrature (areas under curves). In 'Arithmetica Infinitorum',published in 1656, he developed the standard notation for powers, extending the notation (and rules of multiplication) from positive integers to negative integers, zero, and rational numbers. Then he went on to extend Cavalieri's quadrature formula to rational exponents and negative exponents (except -1). But he was unable to find the area under the circular curve y = sqrt(1 - x^2) by applying that area formula for powers of x, because he did not have a way of writing that square root of a binomial as a sum of powers of x. It was Newton who later came up with a generalization of the binomial theorem to exponents that are rational numbers (like one-half) --- as well as exponents that are negative numbers. Then Newton was able to devise a sum (an infinite series) for the area under a circle (or its quadrant), and thus a series for pi. Wallis was able to devise an infinite product for pi --- one that was an improvement over the infinite product that was found earlier by Vieta (see above). The Wallis product had the advantage that it was easier to compute because it involved only integers, whereas the Vieta product involved square roots (an infinite number of them). Sir Jonas Moore (1617 to 1679) was an English mathematician, surveyor, Ordnance Officer and patron of astronomy. In later life, his wealth and influence as Surveyor General of the Ordnance enabled him to become a patron and principal driving force behind the establishment of the Royal Observatory, Greenwich. By 1650 he was an established mathematics teacher and published his first book, 'Moores Arithmetick'. In the 1660's, he was involved in some large scale surveying projects. Henry Oldenberg (1618 to 1677) was born in Bremen, Germany. He trained in theology. He came to London in 1653, as a diplomat and settled in England where his lifelong patron was Robert Boyle. He became a 'natural philosopher'. After the Restoration, he became an early member (original fellow) of the Royal Society (founded in 1660), and served as its first secretary along with John Wilkins, maintaining an extensive network of scientific contacts through Europe. He also became the founding editor of the 'Philosophical Transactions of the Royal Society'. Oldenburg began the practice of sending submitted manuscripts to experts who could judge their quality before publication. This was the beginning of both the modern scientific journal and the practice of peer review. 'Philosophical Transactions of the Royal Society' continues today and is the longest running scientific journal in the world. Michelangelo Ricci (1619 to 1682) was an Italian mathematician and a Cardinal of the Roman Catholic Church. He studied mathematics under Benedetto Castelli who had been a student of Galileo Galilei. Ricci studied the maxima of functions of the form x^m * (a - x)^n and tangents to curves with equation y^m = k * x^n, using methods that are an early form of induction. He also studied spirals (1644) and cycloids (1674) and recognised that the study of tangents and the calculation of areas are reciprocal operations (anticipating the derivatives and anti-derivatives of the soon to be developed calculus). Ricci is also known for his correspondence with Torricelli, Vincenzo Viviani, and Rene de Sluze (listed on this page). William Brouncker (1620? to 1684) was an English mathematician and the first President of the Royal Society. His mathematical work concerned in particular the calculations of the lengths of the parabola and cycloid, and the quadrature of the hyperbola, which requires approximation of the natural logarithm function by infinite series. He was the first in England to take interest in generalized continued fractions and, following the work of John Wallis, he provided development in the generalized continued fraction of pi. Nicolaus Mercator (1620? to 1687) --- not to be confused with Gerardus Mercator the cartographer. Born in Germany, Nicolaus taught mathematics in London (1658-1682). In 1666 he was elected a Fellow of the Royal Society. He designed a marine chronometer for Charles II, and designed and constructed the fountains at the Palace of Versailles (1682-1687). Mathematically, he is most well known for his treatise 'Logarithmo-technica' on logarithms, published in 1668. Abbe Jean Picard (1620 to 1682) was a French astronomer and priest. He was the first person to measure the size of the Earth to a reasonable degree of accuracy in a survey conducted in 1669-70. Picard was the first to attach a telescope with crosswires (developed by William Gascoigne) to a quadrant, and one of the first to use a micrometer screw on his instruments. The sextant he used to find the meridian had a radius of six feet, and was equipped with a micrometer to enable minute adjustments. These equipment improvements made the margin of error only ten seconds, as opposed to Tycho Brahe's four minutes of error. This made his measurements 24 times as accurate. Isaac Newton was to use this level of accuracy in his theory of universal gravitation. Picard collaborated and corresponded with many scientists, including Isaac Newton, Christiaan Huygens, Ole Rømer, Rasmus Bartholin, Johann Hudde, and even his main competitor, Giovanni Cassini. Picard also developed what became the standard method for measuring the right ascension of a celestial object. In this method, the observer records the time at which the object crosses the observer's meridian. Picard made his observations using the precision pendulum clock that Dutch physicist Christiaan Huygens had recently developed. Claude François Milliet Dechales (1621 to 1678), a French Jesuit, is best remembered for 'Cursus seu mundus mathematicus' published in Lyons in 1674, a complete course of mathematics. Topics covered in this wide ranging work included practical geometry, mechanics, statics, magnetism and optics as well as topics outside the usual topics of mathematics such as geography, architecture, astronomy, natural philosophy and music. Rene Francois de Sluse (1622 to 1685) was a Walloon (Belgian) mathematician and churchman. His correspondents included Blaise Pascal, Christiaan Huygens, John Wallis, and Michelangelo Ricci. He was elected a Fellow of the Royal Society in 1674. Sluse contributed to the development of calculus and this work focuses upon spirals, tangents, turning points and points of inflection. He and Johannes Hudde found algebraic algorithms for finding tangents, minima and maxima that were later utilized by Isaac Newton. He is described by John Wallis in his 'Algebra' as "a very accurate and ingenious person." Several of his works were included in the 'Transactions of the Royal Society', for example, his method of drawing tangents to geometrical curves. Vincenzo Viviani (1622 to 1703) was an Italian mathematician and scientist. He was a pupil of Torricelli and a disciple of Galileo. In 1639, at the age of 17, he was an assistant of Galileo Galilei in Arcetri. He remained a disciple until Galileo's death in 1642. From 1655 to 1656, Viviani edited the first edition of Galileo's collected works. In 1660, Viviani and Giovanni Alfonso Borelli conducted an experiment to determine the speed of sound. Timing the difference between the seeing the flash and hearing the sound of a cannon shot at a distance, they calculated a value of 350 meters per second (m/s), considerably better than the previous value of 478 m/s obtained by Pierre Gassendi. In 1737, the Church finally allowed Galileo to be reburied in a grave with an elaborate monument. The monument that was created in the church of Santa Croce was constructed with the help of funds left by Viviani for that specific purpose. Viviani's own remains were moved to Galileo's new grave as well. (Another interesting 'death story'.) John Newton (1622 to 1678) was an English mathematician and astronomer. He was the author of several works on arithmetic and astronomy, designed to facilitate the use of decimal notation and logarithmic methods. Blaise Pascal (1623 to 1662), a French mathematician, physicist, inventor, writer and Christian philosopher. At just 21 years of age, he designed a machine that could add and subtract. It was efficient enough to be commercially produced, under the name of a Pascaline. He made important contributions to the study of fluids, and clarified the concepts of pressure and vacuum by generalising the work of Evangelista Torricelli. He wrote a significant treatise on the subject of 'projective geometry' at the age of 16, and later corresponded with Pierre de Fermat on probability theory, strongly influencing the development of modern economics and social science. Following Galileo Galilei and Torricelli, in 1646, he rebutted Aristotle's followers who insisted that nature abhors a vacuum. Pascal's results caused many disputes before being accepted. Between 1658 and 1659 he wrote on the cycloid and its use in calculating the volume of solids. Pascal had poor health, especially after the age of 18, and he died just two months after his 39th birthday. Note: Pascal's triangle was known for many centuries before Pascal himself lived. In China it is known as Yanghui's triangle; in Iran as Khayyam's triangle (see Khayyam above); in Italy as Tartaglia's triangle. This triangle of integers arises in expanding binomials, like (x + y)^n. Pascal was the first to use the triangle of integers to calculate probabilities. Stefano degli Angeli (1623 to 1697) was born in Venice and was a Gesuati mathematician. He was entrusted to publish Cavalieri's final work, 'Exercitationes geometricae sex', since by 1647 Cavalieri's health had deteriorated to such an extent that he was unable to carry out the work himself. Angeli also corresponded with a number of other mathematicians including Torricelli and Viviani. Angeli's many mathematical works were on infinitesimals and he used them to study spirals, parabolas and hyperbolas. Angeli examined fluid statics based on Archimedes' principle and Torricelli's experiments. He also considered the motion of bodies falling towards a rotating Earth. Angeli often refers to Galileo in his writings on physics, showing clearly how he has been influenced, particularly in terms of ways of approaching problems via the experimental method. Also clearly influenced by Galileo is Angeli's writings on the two systems of Ptolemy and Copernicus which he writes in Galileo's dialogue style. Jean-Baptiste du Hamel (1624 to 1706) was a French cleric and natural philosopher, and the first secretary of the 'Academie Royale des Sciences'. He was sent to England to assist a French diplomat, and while there, met with Robert Boyle, Henry Oldenburg, and other members of the Royal Society. His scientific works were published between 1642 and 1673. In his later years, Du Hamel began to devote himself to his religious work, stepping back from scientific studies. John Collins (1625 to 1683) was an English mathematician. He is most known for his extensive correspondence with leading scientists and mathematicians such as Giovanni Alfonso Borelli, Gottfried Leibniz, Isaac Newton, and John Wallis. His correspondence provides details of many of the discoveries and developments made in his time, and shows his activity as an 'intelligencer'. To him was due the printing of Isaac Barrow's 'Optical and Geometrical Lectures', as well as of his editions of 'Apollonius' and 'Archimedes'; of John Kersey's 'Algebra', Thomas Branker's translation of Rhonius's 'Algebra', and Wallis's 'History of Algebra'. He took an active part in seeing Jeremiah Horrocks's 'Astronomical Remains' through the press. Collins' books and papers included a voluminous correspondence with Newton, Leibniz, Gregory, Barrow, John Flamsteed, Wallis, Slusius, and others. Pietro Mengoli (1625 to 1686) was an Italian mathematician and clergyman from Bologna, where he studied with Bonaventura Cavalieri at the University of Bologna, and succeeded him in 1647. He remained as professor there for the next 39 years of his life. In 1644, it was Mengoli who first posed the famous Basel problem, solved in 1735 by Leonhard Euler. He wrote a paper in 1650 in which he proved that the sum of the alternating harmonic series is equal to the natural logarithm of 2. He also proved that the harmonic series does not converge, and provided a proof that Wallis' infinite product for pi is correct. Erhard Weigel (1625 to 1699) was a German mathematician, astronomer and philosopher. He was the teacher of Leibniz in summer 1663, and other notable students. He also worked to make science more widely accessible to the public, and what would today be considered a 'populariser' of science. Jean Dominique Cassini (Giovanni Domenico Cassini) (1625 to 1712) was an Italian mathematician, astronomer, astrologer and engineer. Cassini discovered four satellites of the planet Saturn and noted the division of the rings of Saturn; the Cassini Division was named after him. The Cassini spaceprobe, launched in 1997, was named after him and became the fourth to visit Saturn and the first to orbit the planet. Cassini observed and published surface markings on Mars (earlier seen by Huygens but not published) and determined the rotation periods of Mars and Jupiter. He shares with Robert Hooke credit for the discovery of the Great Red Spot on Jupiter (ca. 1665). Around 1690, Cassini was the first to observe differential rotation within Jupiter's atmosphere. In 1672 he sent his colleague Jean Richer to Cayenne, French Guiana, while he himself stayed in Paris. The two made simultaneous observations of Mars and, by computing the parallax, determined its distance from Earth. This allowed for the first time an estimation of the dimensions of the solar system: since the relative ratios of various sun-planet distances were already known from geometry, only a single absolute interplanetary distance was needed to calculate all of the planet distances to the sun. Cassini initially held the Earth to be the centre of the solar system, though later observations compelled him to accept the model of the solar system proposed by Tycho Brahe, and eventually that of Nicolaus Copernicus. Giovanni Domenico Cassini was also the first of his family to begin work on the project of creating a topographic map of France. The project was continued by his son Jacques Cassini and eventually finished by his grandson César-François Cassini de Thury . Johannes Hudde (1628 to 1704) was a burgomaster (mayor) of Amsterdam between 1672 - 1703, a mathematician, and governor of the Dutch East India Company. Hudde corresponded with Baruch Spinoza and Christiaan Huygens, Johann Bernoulli, Isaac Newton and Leibniz. Newton and Leibniz mention Hudde many times and used some of his ideas in their own work on infinitesimal calculus. Christian_Huygens (1629 to 1695) was a prominent Dutch mathematician and scientist. He is known particularly as an astronomer, physicist, probabilist and horologist (clock designer-maker). His work included early telescopic studies of the rings of Saturn and the discovery of its moon Titan, the invention of the pendulum clock and other investigations in timekeeping. He published major studies of mechanics and optics, and pioneered work on games of chance. Isaac Barrow (1630 to 1677) was an English Christian theologian and mathematician who is generally given credit for his early role in the development of infinitesimal calculus; in particular, for the discovery of the fundamental theorem of calculus. His work centered on the properties of the tangent. Isaac Newton was a student of Barrow's, and Newton went on to develop calculus in a more modern form. John Locke (1632 to 1704) was an English philosopher and physician, widely regarded as one of the most influential of Enlightenment thinkers. Considered one of the first of the British empiricists, following the tradition of Sir Francis Bacon, he is equally important to social contract theory. His writings influenced Voltaire and Jean-Jacques Rousseau, many Scottish Enlightenment thinkers, as well as the American revolutionaries. His contributions to social contract theory are reflected in the United States Declaration of Independence. Such was Locke's influence that Thomas Jefferson wrote: "Bacon, Locke and Newton ... I consider them as the three greatest men that have ever lived, without any exception, and as having laid the foundation of those superstructures which have been raised in the Physical and Moral sciences". Christopher Wren (1632 to 1723) is one of the most highly acclaimed English architects in history. Wren was a notable anatomist, astronomer, geometer, and mathematician-physicist, as well as an architect. He was a founder of the Royal Society (president 1680-82), and his scientific work was highly regarded by Isaac Newton and Blaise Pascal. Hendrik van Heuraet (1633 to 1660?) was a Dutch mathematician. From 1653, he studied at Leiden University where he interacted with Frans van Schooten, Johannes Hudde, and Christiaan Huygens. He did some work on recification of curves. Petrus van Schooten (1634 to 1679) was a fortress architect. Robert Hooke (1635 to 1703) was an English natural philosopher and architect. At one time he was simultaneously the curator of experiments of the Royal Society, a member of its council, Gresham Professor of Geometry, and Surveyor to the City of London after the Great Fire of London (in which capacity he appears to have performed more than half of all the surveys after the fire). Robert Gunther's 'Early Science in Oxford', a history of science in Oxford during the Protectorate, Restoration and Age of Enlightenment, devotes five of its fourteen volumes to Hooke. Hooke made contributions in Mechanics, Gravitation, Horology, Microscopy, Palaeontology, Astronomy, and Memory. James Gregory (1638 to 1675) was a Scottish mathematician and astronomer. He described an early practical design for the reflecting telescope - the Gregorian telescope - and made advances in trigonometry, discovering infinite series representations for several trigonometric functions. Nicolas Malebranche (1638 to 1715) was a French Oratorian and rationalist philosopher. Malebranche wrote on the laws of motion, a topic he discussed extensively with Leibniz. He also wrote on mathematics and, although he made no major mathematical discoveries of his own, he was instrumental in introducing and disseminating the contributions of Descartes and Leibniz in France. Malbranche introduced l'Hôpital to Johann Bernoulli, with the ultimate result being the publication of the first textbook in infinitesimal calculus. Francois de Raynaud (Regnauld) (ca. 1670) was a student of Honore Fabri (see above, near 1606 births) who tried to unify all physics along the lines of geometry. . Jacques Ozanam (1640 to 1718) was a French mathematician. He gave up theology after four years of study and began to give free private instruction in mathematics at Lyon. In 1670, he published trigonometric and logarithmic tables more accurate than the existing ones of Ulacq, Pitiscus, and Briggs. His mathematical publications were numerous and well received. 'Récréations mathématiques et physiques' (published 1694) was later translated into English and is well known today. He was elected a member of the Académie des Sciences in 1701. Seki Takakazu (1642 to 1708) was a Japanese mathematician. He has been described as Japan's "Newton." He created a new algebraic notation system, and also, motivated by astronomical computations, did work on infinitesimal calculus and Diophantine equations. A contemporary of Gottfried Leibniz and Isaac Newton, Seki's work was independent. He was born the same year as Newton. Isaac Newton (1642 to 1727) is known for developing/discovering/elucidating differential calculus integral calculus the Fundamental Theorem of Calculus, which shows the relationship between differentiation and integration the 3 'Newtonian' laws of motion the law of gravity --- any two bodies in the universe attract each other as the inverse of the square of the distance apart (of the centers) the precession of the equinoxes

This is the kind of argument that Newton went through
to show that Kepler's observation was derivable from
the fact that gravitational attraction falls off as
the radius (distance between centers) squared.

Kepler and Newton --- both geniuses.

These logarithmic graphs demonstrate the
T^2 = K * R^3 relationship,
or
2 * log T = 3 * log R + log K
or
log R = (2/3) * log T - (log K / 3)

In other words, these graphs show the
linear relationship between log T and log R.

Olaus Roemer (1644 to 1710) was a Danish astronomer who in 1676 made the first quantitative measurements of the speed of light. Gottfried Leibniz (1646 to 1716), a German mathematician, designed a machine that could perform the four basic arithmetic operations - addition, subtraction, multiplication, and division. He was therefore rather scornful of the limited functions of the Pascaline (see Pascal above). Unfortunately, the carry mechanism was faulty and, hence, the machine was never produced in quantity. Leibniz is known as the co-discoverer or independent discoverer of the 'infinitesimal' calculus --- along with Isaac Newton. Leibniz published first. Leibniz contributed a fair amount to the statics and dynamics emerging around him, often disagreeing with Descartes and Newton. He devised a new theory of motion (dynamics) based on kinetic energy and potential energy, which posited space as relative, whereas Newton was thoroughly convinced that space was absolute. An important example of Leibniz's mature physical thinking is his 'Specimen Dynamicum' of 1695. Leibniz's 'vis viva' (Latin for "living force") is m*v^2, twice the modern kinetic energy. He realized that the total energy would be conserved in certain mechanical systems, so he considered it an innate motive characteristic of matter. Here too his thinking gave rise to another regrettable nationalistic dispute. His 'vis viva' was seen as rivaling the conservation of momentum championed by Newton in England and by Descartes in France; hence academics in those countries tended to neglect Leibniz's idea. In reality, both energy and momentum are conserved, so the two approaches are equally valid. John Flamsteed (1646 to 1719) was an English astronomer and the first astronomer Royal. He catalogued over 3000 stars. His star catalogue tripled the number of entries in Tycho Brahe's sky atlas. Denis Papin (1647 to 1712) was a French physicist, mathematician and inventor, best known for his pioneering invention of the steam digester, the forerunner of the steam engine, and of the pressure cooker. Giovanni Ceva (1647 to 1734) was an Italian mathematician widely known for proving Ceva's theorem in elementary geometry. But it was proven much earlier by Yusuf Al-Mu'taman ibn Hud, an eleventh-century king of Zaragoza. Giovanni Ceva also studied applications of mechanics and statics to geometric systems. Ceva also worked on hydraulics. In 1728, he published 'Opus hydrostaticum' which discusses his work in hydraulics. In fact, he used his knowledge of hydraulics to stop a project from diverting the river Reno into the river Po. Tommaso Ceva (1648 to 1737) was an Italian mathematician. He was the brother of Giovanni Ceva. His only mathematical work, published in 1699, was the 'Opuscula Mathematica' which dealt with geometry, gravity and arithmetic. Joseph Raphson (1648? to 1715?) was an English mathematician known best for the Newton-Raphson method of approximating roots of equations. He was made a Fellow of the Royal Society in 1689, after being proposed for membership by Edmund Halley. Raphson was a staunch supporter of Newton's claim, and not that of Gottfried Leibniz, to be the sole inventor of calculus. In addition, Raphson translated Newton's 'Arithmetica Universalis' into English. Count E.W. von Tschirnhaus (1651 to 1708) was a German mathematician, physicist, physician, and philosopher. In 1696, Johann Bernoulli posed the problem of the brachystochrone to the readers of 'Acta Eruditorum'. Tschirnhaus was one of only five mathematicians to submit a solution. Bernoulli published these contributions (including Tschirnhaus') along with his own in the journal in May of the following year. In 1682, Von Tschirnhaus worked out the theory of catacaustics (optic reflections) and showed that they were rectifiable (had finite length). This was the second case in which the envelope of a moving line was determined. One of the catacaustics of a parabola still is known as Tschirnhausen cubic. Von Tschirnhaus produced various types of lenses and mirrors, some of them are displayed in museums. He erected a large glass works in Saxony, where he constructed burning glasses of unusual perfection and carried on his experiments (1687-1688). Joseph Sauveur (1653 to 1716) was a French mathematician and physicist. He was a professor of mathematics and in 1696 became a member of the French Academy of Sciences. Sauveur did work on hydrostatics. Sauveur is known principally for his detailed studies on acoustics. Due to a hearing and speech impairment, musicians served as his ears and voices. His work included researching the correlation between frequency and musical pitch. He has been credited with coining the term acoustique, which he derived from an ancient Greek word meaning "able to be heard". Pierre Varignon (1654 to 1742) was a French mathematician. Varignon was a friend of Newton, Leibniz, and the Bernoulli family. Varignon's principal contributions were to graphic statics and mechanics. He simplified the proofs of many propositions in mechanics, adapted Leibniz's calculus to the inertial mechanics of Newton's Principia, and treated mechanics in terms of the composition of forces in 'Projet d'une nouvelle mécanique' in 1687. Among Varignon's other works was a 1699 publication concerning the application of differential calculus to fluid flow and to water clocks. In 1690, he created a mechanical explanation of gravitation. In 1702, he applied calculus to spring-driven clocks. In 1704, he invented the U-tube manometer, a device capable of measuring rarefaction in gases. He was elected to the Académie Royale des Sciences in 1688. He was elected to the Berlin Academy in 1713 and to the Royal Society in 1718. The Bernoulli family, over the course of three generations (ca. 1654 to 1807) produced eight mathematically gifted academics who, among them, contributed to the foundations of applied mathematics and physics. Jacob (or Jacques) Bernoulli (1654 to 1705) was a Swiss mathematician. He is known for his numerous contributions to calculus, and along with his brother Johann (born 1667), was one of the founders of the calculus of variations. He also discovered the fundamental mathematical constant 'e'. However, his most important contribution was in the field of probability, where he derived the first version of the 'law of large numbers' in his work 'Ars Conjectandi'. Edmond Halley (ca. 1656 to 1742) was an English astronomer, geophysicist, mathematician, meteorologist, and physicist who is best known for computing the orbit of Halley's Comet. He was the second Astronomer Royal in Britain, succeeding John Flamsteed. Halley is also known to be interested in the nature of gravity, along with Robert Hooke and Christopher Wren. Halley went to Newton in Cambridge and found that Newton had mathematical proofs of Kepler's laws of planetary motion. He convinced Newton to publish his results in a short treatise which is often called 'De Motu'. Newton eventually expanded 'De Motu' into the 3-book 'Principia'. Halley provided the funds to print it.

These images show that lunar and solar
eclipses occur when the moon crosses
crosses into the 'ecliptic plane' ---
the plane of the Earth's orbit.

THE LINKS BELOW ARE 'UNDER CONSTRUCTION'.

Some may be removed. Some will have notes added.

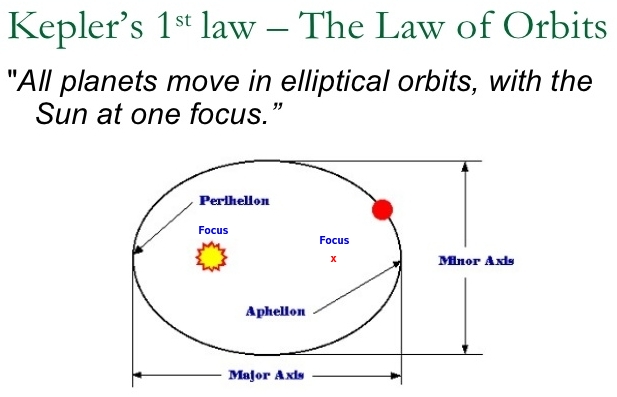
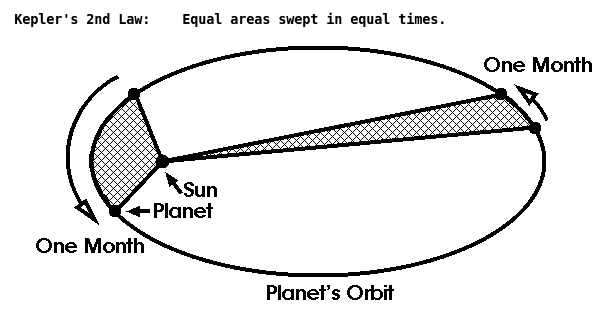
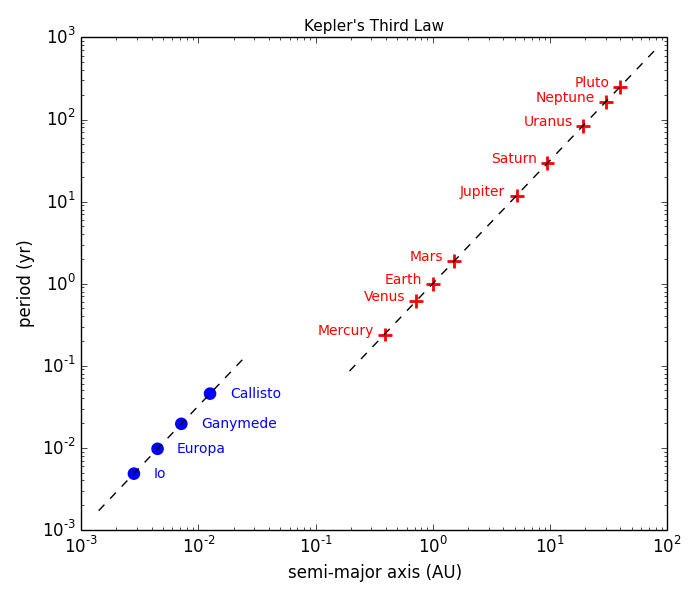
Charles Renee Reynaud (1656 to 1728) Bernard de Fontanelle (1657 to 1757) Jean Christophe Fatio (1659 to 1720) David Gregory (1659 to 1708) was a Scottish mathematician and astronomer. He was professor of mathematics at the University of Edinburgh, Savilian Professor of astronomy at the University of Oxford, and a commentator on Isaac Newton's Principia. He was a nephew of astronomer and mathematician James Gregory. Marquis Guillaume de L'Hospital (1661 to 1704) Textbook on differential calculus ~1696 Louis Carre (1663 to 1711) was a French mathematician and member of the French Academy of Sciences. He was the author of one of the first books on integral calculus. John Craig (1663 to 1731 ca. 1687) was a Scottish mathematician and theologian. Takebe Kenko (or Takebe Katahiro) (1664 to 1739) Nicolas Fatio de Duiller (1664 to 1753) Antoine Parent (1666 to 1716) was a French mathematician, born at Paris and died there, who wrote in 1700 on analytical geometry of three dimensions. His works were collected and published in three volumes at Paris in 1713. John Harris (ca. 1666 to 1719) Abraham de Moivre (1667 to 1754) Jean Bernoulli (Johann) (1667 to 1748) Giovanni Girolamo Saccheri (1667 to 1733) was an Italian Jesuit priest, scholastic philosopher, and mathematician. Luigi Guido Grandi (1671 to 1742) was an Italian monk, priest, philosopher, mathematician, and engineer. George Cheyne (1671-1743) was a pioneering physician, early proto-psychologist, philosopher and mathematician. John Keill (1671 to 1721) was born in Edinburgh, Scotland, and was primarily a mathematician and a disciple of Isaac Newton. He studied at Edinburgh University, under David Gregory, and obtained his bachelors degree in 1692 with a distinction in physics and mathematics. William Jones (1675 to 1749) was a Welsh mathematician, most noted for his proposal for the use of the Greek letter pi to represent the ratio of the circumference of a circle to its diameter. He was a close friend of Sir Isaac Newton and Sir Edmund Halley. In November, 1711 he became a Fellow of the Royal Society, and was later its Vice-President. Humphry Ditton (1675 to 1715) was an English mathematician. He studied theology, but on the death of his father, he devoted himself to the study of mathematics. Through the influence of Isaac Newton he was elected mathematical master in Christ's Hospital. He did work on perspective. Jacopo Riccati (1676 to 1754) was an Italian mathematician, born in Venice. He is now remembered for the Riccati equation. Antonio Schinella Conti (1677 to 1749) was an Italian historian, mathematician, philosopher and physicist. He was known as Abbé Conti (in Italian, Abate Conti) and is famous for having been the intermediary, in England in 1715-16, in the Leibniz-Newton calculus controversy. Jacques Cassini (1677 to 1756) was a French astronomer, son of the famous Italian astronomer Giovanni Domenico Cassini. Pierre Rémond de Montmort (1678 to 1719) was a French mathematician. De Montmort is known for his book on probability and games of chance. Another of de Montmort's interests was the subject of finite differences. Charles Hayes (1678-1760) was an English mathematician and chronologist, author of an early book on the method of fluxions. Jakob Hermann (1678 to 1733) was a mathematician who worked on problems in classical mechanics. He appears to have been the first to show that the Laplace-Runge-Lenz vector is a constant of motion for particles acted upon by an inverse-square central force. He received his initial training from Jacob Bernoulli and was a distant relative of Leonhard Euler. Ephraim Chambers (ca. 1680 to 1740) Wrote 'Cyclopaedia'. John Colson (1680 to 1760) Translated several of Newton's works into English. Gabriele Manfredi (1681 to 1761) was an Italian mathematician who undertook important work in the field of calculus. Roger Cotes (1682 to 1716) was an English mathematician, known for working closely with Isaac Newton by proofreading the second edition of his famous book, the Principia, before publication. He also invented the quadrature formulas known as Newton-Cotes formulas and first introduced what is known today as Euler's formula. He was the first Plumian Professor at Cambridge University from 1707 until his death. Count Giulio Carlo di Fagnano (1682 to 1766) was an Italian mathematician. He was probably the first to direct attention to the theory of elliptic integrals. Amédée-François Frézier (1682 to 1773) Savin (ca. 1701) (need info and link) Guisnee (ca. 1705) (need info and link) Chamberlayne (ca. 1718) (need info and link) Burkhardt (ca. 1721) (need info and link) Bragelonge (ca. 1730) (need info and link) Brook Taylor (1685 to 1731), an English mathematician, coined the phrase "vanishing point". His treatise 'Linear Perspective' (1715) expounded the principle of vanishing points and was of value to artists. This is the same Taylor who originated Taylor's theorem, a formula important in differential calculus, which relates a function to its derivatives by means of a power series. Nicolaus I Bernoulli (1687 to 1759) Robert Simson (1687 to 1768 ca. 1756) A Scottish mathematician and professor of mathematics at the University of Glasgow. In 1756, appeared, both in Latin and in English, the first edition of his Euclid's 'Elements'. This work, which contained only the first six and the eleventh and twelfth books, and to which, in its English version, he added the Data in 1762, was for long the standard text of Euclid in England. Christlieb von Clausberg (1689 to 1751) Died in Copenhagen. (need more info and link) Heinrich? Kuhn (1690? to 1769?) A teacher in Danzig. In 1750, was the first to give the square root of minus one a geometric picture, analogous to the geometric interpretation of the negative reals. (need more info and a link) Mentioned in 'A History of Mathematics' by Cajori. James Stirling (1692 to 1770) was a Scottish mathematician. The Stirling numbers, Stirling permutations, and Stirling's approximation are named after him. He also proved the correctness of Isaac Newton's classification of cubics Henry Pemberton (1694 to 1771) was an English physician and man of letters. He became Gresham Professor of Physic, and edited the third edition of Principia Mathematica. Voltaire (1694 to 1778) Nicolaus II Bernoulli (1695 to 1726) Henri Pitot (1695 to 1771) was a French hydraulic engineer and the inventor of the pitot tube. The Pitot theorem of plane geometry is named after him. Colin MacLaurin (1698 to 1746) was a Scottish mathematician. The Maclaurin series, a special case of the Taylor series, is named after him. George Campbell (ca. 1700 to 1766) Involved in a dispute with Colin MacLaurin (1698 - 1746) over complex roots. Samuel Klingenstierna (1698 to 1765) Swedish mathematician Maupertuis (1698 to 1759) Often credited with having invented the principle of least action. Pierre Bonger (1698 to 1758) (need more info and link) Daniel Bernoulli (1700 to 1782) William Braikenridge (also Brakenridge) (1700? to 1762) was a Scottish mathematician and cleric, a Fellow of the Royal Society from 1752.In geometry the Braikenridge-Maclaurin theorem was independently discovered by Colin Maclaurin. It occasioned a priority dispute after Braikenridge published it in 1733. Edmund Stone (ca. 1700 to 1768) Son of a gardener of the Duke of Argyll. Self-taught. Charles Marie de la Condamine (1701 to 1774) Thomas Bayes (1701 to 1761) An English mathematician and Presbyterian minister, known for having formulated a specific case of the theorem that bears his name: Bayes's theorem. His friend Richard Price edited and presented this work in 1763, after Bayes' death, as 'An Essay towards solving a Problem in the Doctrine of Chances'. Georg Wolfgang Krafft (1701 to 1754) When Euler became the professor of mathematics in St. Petersburg in 1733, Krafft took over Euler's previous position, that of professor of physics. Gabriel Cramer (1704 to 1752) Swiss mathematician --- namesake of Cramer's rule. Louis Goudin (1704 to 1760) A French astronomer and member of the French Academy of Sciences. He worked in Peru, Spain, Portugal and France. Johann Andreas Segner (1704 to 1777) Was a Hungarian-born scientist. He was born in the Kingdom of Hungary, in the former Hungarian capital city of Pozsony (today Bratislava). In 1735, Segner became the first professor of mathematics at the University of Göttingen, a position created for him. One of the best-known scientists of his age, Segner was a member of the academies of Berlin, London, and Saint Petersburg. Segner produced the first proof of Descartes' rule of signs. He was the first scientist to use the reactive force of water and constructed the first water-jet, the Segner wheel, which resembles one type of modern lawn sprinkler. Historians of science remember him as the father of the water turbine. Jean Castillon [aka Giovanni Salvemini] (1704-1791) Was as an Italian mathematician and astronomer. In 1745, he was elected to the Royal Society. In 1765, Frederick the Great appointed him 'astronomer Royal' of the Observatory of Berlin. Succeeding Joseph-Louis Lagrange, he was appointed Director of the Mathematics Section of the Berlin Academy, a role he held until his death. He studied conic sections, cubic equations and problems of artillery. Among his latest publications mathematics note He is also known for the "Castillon's problem". Leonhard Euler (1707 to 1783) - super prolific mathematician. solved the 7 bridges of Konigsberg problem --- led to graph theory V + F = E + 2 --- the formula connecting the vertices, faces, and edges of a solid plyhedron. The number 'e' is such that the gradient of y=e^x is equal to itself. e^(i x pi) = -1 Vincenzo Riccati (1707 to 1775) Giordano Riccati (1709 to 1790) Eustachio Zanotti (1709-1782) Was a noted astronomer and mathematician and was the son of Giampietro Zanotti, an Italian painter and art historian. (need a better link) Thomas Simpson (1710 to 1761) Wrote popular textbooks, including works on 'fluxions'. Patrick_Murdoch (ca. 1710? to 1774) Part of Murdoch's 1746 'Newtoni genesis curvarum per umbras' (Newton's generation of curves by shadows) deals with perspective. François Jacquier (1711 to 1788) A French priest. In 1739-42, François Jacquier and another French priest, Thomas LeSeur (17xx? to 17xx?), produced, with the assistance of Jean-Louis Calandrini (1703-1758), an extensively annotated version of the 1726 3rd edition of Newton's 'Principia'. Sometimes this is referred to as the 'Jesuit edition'. It was much used, and reprinted more than once in Scotland during the 19th century. David Hume (1711 to 1776) Scottish philosopher, historian, economist. Johann Samuel König (1712 to 1757) Had disagreements with Euler on the principle of least action. Christian Ehrenfried Eschenbach (1712-1788) belongs to the forerunners of scholars of legal medicine in Germany. As a principal re-elected 11 times and dean of the medical faculty at Rostock University, he defended academic positions in difficult times. His bibliography comprises numerous text books, e.g. on surgery, anatomy, pathology and obstetrics as well as various fields of mathematics. Alexis Claude Clairaut (1713 to 1765) See Clairaut's theorem and Clairaut's equation and Clairaut's relation. Jean Paul de Gua (1713 to 1785) A French mathematician who published in 1740 a work on analytical geometry in which he applied it, without the aid of differential calculus, to find the tangents, asymptotes, and various singular points of an algebraic curve. Denis Diderot (1713 to 1784) Best known for serving as co-founder, chief editor, and contributor to the Encyclopédie along with Jean le Rond d'Alembert (below). First volume was published in 1751. Cesar Francois Cassini (1714 to 1784) French astronomer-surveyor-cartographer. Arima Yoriyuki (1714 to 1783) Japanese mathematician. In 1766, he found the a rational approximation of pi, correct to 29 digits --- the ratio of two 15 digit integers. Barmann (ca. 1745) (more info and link needed) Jean d'Alembert (1717 to 1783) French mathematician, mechanician, physicist, philosopher, and music theorist. D'Alembert's formula for obtaining solutions to the wav equation is named after him. The wave equation is sometimes referred to as D'Alembert's equation. Francesco Riccati (1718 to 1791) A son of Jacopo Ricatti (above). Italian architect. (more info and better link needed) Maria Gaetana Agnesi (1718 to 1789) An Italian mathematician and philosopher. She is credited with writing the first book discussing both differential and integral calculus and was an honorary member of the faculty at the University of Bologna. Matthew Stewart (1719 to 1785) Published Stewart's theorem in 1746. Abraham Gotthelf Kastner (1719 to 1800) German mathematician known for his textbooks. John Landen (1719 to 1790 ca. 1771) An English mathematician. Landen's capital discovery is that of the Landen's transformation for the expression of the arc of an hyperbola in terms of two elliptic arcs. His researches on elliptic functions are of considerable elegance, but their great merit lies in the stimulating effect which they had on later mathematicians. He also showed that the roots of a cubic equation can be derived by means of the infinitesimal calculus. He lived a very retired life, and saw little or nothing of society. When he did mingle in it, his dogmatism and pugnacity caused him to be generally shunned. Fridericus Guilielmus De Oppel (1720 to 1769) Wrote 'Analysis Triangulorum' (1746). (need more info and link) Joseph Torelli (1721 to 1781) was an Italian mathematician. His edition of the collected works of Archimedes was printed at Oxford in 1792. The preparation of this work was a labour performed, among many other pursuits, during most of his life. Ravelli (ca. 1751) (need more info and a link) John Lawson (1723 to 1779) An English mathematician. Published some works on tangencies. Jean-Étienne Montucla (1725 to 1799) Wrote a history of math. The first part was published in 1758. Johann Heinrich Lambert (1728 to 1777) A Swiss mathematician, physicist, philosopher and astronomer. He is best known for proving the irrationality of pi. Fredric Mallet (1728 to 1797) Was one of Klingenstierna's (above, 1698) most devoted students. Sorted through Klingenstierna's manuscripts for eventual publication. (need a better link) Charles Bossut (1730 to 1814) Was a French mathematician. His works include 'Traité élémentaire d'hydrodynamique' in 1771, 'Traité élémentaire de méchanique statique' in 1772, 'Cours de mathématiques' in 1781, and 'Histoire générale des mathématiques' in 1810. Étienne Bezout (1730 to 1783) A French mathematician. Wrote 'Théorie générale des équations algébriques', published at Paris in 1779, which, in particular, contained much new and valuable matter on the theory of elimination and symmetrical functions of the roots of an equation. He used determinants in a paper in the 'Histoire de l'académie royale', 1764, but did not treat the general theory. Martin Johan Wallenius (1731 to 1773) All five squarable lunes were given in a dissertation by Martin Johan Wallenius in 1766. (need a better link) Girolamo Saladini (1731-1813) Saladini was a student of Vincenzo Riccati (above), and was a co-author with Riccati of the two volume book 'Institutiones Analyticae' (Vol 1, 1765; Vol 2, 1767) which contains the formulas for the addition and subtraction of hyperbolic functions as well as other, now standard, formulas analogous to those for the circular (sin, cos) functions. They also computed the derivatives of sinh x and cosh x. (need a better link) Gian Francesco Malfatti (1731 to 1807) was an Italian mathematician. He studied in Bologna where his mentors included Vincenzo Riccati, F. M. Zanotti and G. Manfredi. Malfatti posed the problem of carving three circular columns out of a triangular block of marble, using as much of the marble as possible, and conjectured that three mutually-tangent circles inscribed within the triangle would provide the optimal solution. These tangent circles are now known as Malfatti circles after his work, despite the earlier work of Japanese mathematician Ajima Naonobu and of his countryman Gilio di Cecco da Montepulciano on the same problem --- and despite the fact that the conjecture was later proven false. Several triangle centers derived from these circles are also named after both Ajima and Malfatti. Additional topics in Malfatti's research concerned quintic equations, and the property of the lemniscate of Bernoulli that a ball rolling down an arc of the lemniscate, under the influence of gravity, will take the same time to traverse it as a ball rolling down a straight line segment connecting the endpoints of the arc. Francois Daviet de Foncenex (1734 to 1799) In 1759, he attempted a proof of the fundamenatal theorem of algebra --- as did Euler in 1749, Lagrange in 1772, and Laplace in 1795. (need a better link) Achille_Pierre Dionis du Sejour (1734 to 1794) was a French astronomer and mathematician. He wrote on algebraic curves as well as on astronomy (planets and comets). William Wales (1734? to 1798) Made astronical observations and calculations on the 2nd voyage of Captain James Cook. Alexandre-Théophile Vandermonde (1735 to 1796) A French musician (violinist), mathematician and chemist who worked with Bézout and Lavoisier. His name is now principally associated with determinant theory in mathematics. Joseph Louis Lagrange (1736 to 1813) An Italian mathematician and astronomer. He made significant contributions to all fields of analysis, number theory, and both classical and celestial mechanics. In 1766, Lagrange succeeded Euler as the director of mathematics at the Prussian Academy of Sciences in Berlin, Prussia, where he stayed for over twenty years. Edward Waring (1736 to 1798) An English mathematician who was elected in 1760 as Lucasian Professor of Mathematics at Cambridge, holding the chair until his death. In 1762, he published the full 'Miscellanea Analytica', mainly devoted to the theory of numbers and algebraic equations. See Waring's (number theory) problem and Waring's prime number conjecture. Erland Samuel Bring (1736 to 1798) was a Swedish mathematician. At Lund University, he wrote eight volumes of mathematical work in the fields of algebra, geometry, analysis and astronomy, including 'Meletemata quaedam mathematematica circa transformationem aequationum algebraicarum' (1786). Bring developed a transformation to simplify a quintic equation to the form x^5 + px + q = 0. Jacques Antoine Joseph Cousin (1739 to 1800) Wrote 'Lecons de Calcul Differentiel et de Calcul Integral' (1777). His main position was as professor for mathematics and experimental physics at the College Royale, which he held from 1769 until his death. (need a better link) Anders Johan Lexell (1740 to 1784) was a Finnish-Swedish astronomer, mathematician, and physicist who spent most of his life in Russia. Lexell made important discoveries in polygonometry and celestial mechanics. He contributed to spherical trigonometry with new and interesting solutions, which he took as a basis for his research of comet and planet motion. His name was given to a theorem of spherical triangles. Lexell was one of the most prolific members of the Russian Academy of Sciences at that time, having published 66 papers in 16 years of his work there. In later life (circa 1783), Lexell became very attached to Leonhard Euler, who lost his sight in his last years but continued working using his elder son Johann Euler to read for him. Lexell helped Leonhard Euler greatly, especially in applying mathematics to physics and astronomy. He helped Euler to write calculations and prepare papers. Stanislaus Wydra (1741 to 1804) was a professor at Prague University. He wrote an 88 page book titled 'Elementa Calculi Differentialis et Integralis' (1783). He was a pupil, and later biographer, of Joseph Stepling. (Wydra/Vydra needs a better link) Carl Hindenburg (1741 to 1808) was a German mathematician born in Dresden. His work centered mostly on combinatorics and probability. Hindenburg co-founded the first German mathematical journals. Between 1780 and 1800, he was involved at different times with the publishing of four different journals all relating to mathematics and its applications. One of Hindenberg's best students, according to Donald Knuth, is Heinrich August Rothe. Another student, Johann Karl Burckhardt, published the book 'Theorie der Kettenbrüche' after being encouraged by Hindenberg to work on continued fractions. He also influenced Christian Kramp's work in combinatorics. Antonio Cagnoli (1743 to 1816) was an Italian astronomer, mathematician and diplomat. He worked in Paris and Verona. He set up an observatory in Verona --- which was damaged by Bonaparte's cannons, but Bonaparte made amends with money and the gift a very accurate clock. Marie Jean Antoine Nicolas de Caritat, marquis de Condorcet (1743 to 1794) was a French philosopher, mathematician, and early political scientist whose Condorcet method in voting tally selects the candidate who would beat each of the other candidates in a run-off election. Condorcet was one of the first to systematically apply mathematics in the social sciences. In 1786, Condorcet worked on ideas for the differential and integral calculus, giving a new treatment of infinitesimals - a work which was never printed. Caspar Wessel (1745 to 1818) A Norwegian-Danish mathematician and cartographer. In 1799, Wessel was the first person to describe the geometrical interpretation of complex numbers as points in the complex plane. Gaspard Monge (1746 to 1818) Was a French mathematician, the inventor of descriptive geometry (the mathematical basis of technical drawing), and the father of differential geometry. During the French Revolution he served as the Minister of the Marine, and was involved in the reform of the French educational system, helping to found the École Polytechnique. Reuben Burrow (1747 to 1792) An English mathematician and orientalist. Initially a teacher, he was later appointed astronomer-royal at the Royal Greenwich Observatory. He later conducted research in India, becoming one of the first members of the Asiatic Society. He was also interested in ancient geometry, as he has proved by his book on Apollonius: 'A Restitution of the Geometrical Treatise of Apollonius Pergæus on Inclinations' (1779), and was curious to investigate the mathematical treatises in ancient Hindu and other Oriental literature. He later published 'Hindoo Knowledge of the Binomial Theorem'. Jean Dominque IV Cassini (1748 to 1845) French astronomer. D'Amondans Charles de Tinseau (1748 to 1822) Graduated as a military engineer in 1771 and later became a mathematician after becoming a student of Monge (above). ??? Rowing (ca. 1770) (need more info and link) Pierre Simon de Laplace (1749 to 1827) A French mathematician and astronomer whose work was pivotal to the development of mathematical astronomy and statistics. He summarized and extended the work of his predecessors in his five-volume 'Mécanique Céleste' (Celestial Mechanics) (1799-1825). This work translated the geometric study of classical mechanics to one based on calculus, opening up a broader range of problems. In statistics, the Bayesian interpretation of probability was developed mainly by Laplace. In France, the normal distribution is called the Laplacian distribution. (In Germany, it is called the Gaussian distribution.) Lorenzo Mascheroni (1750 to 1800) was an Italian mathematician. In his 'Geometria del Compasso' (1797), he proved that any geometrical construction, which can be done with compass and straightedge, can also be done with compasses alone. However, the priority for this result (now known as the Mohr-Mascheroni theorem) belongs to the Dane Georg Mohr, who had previously published a proof in 1672. In his 'Adnotationes ad calculum integrale Euleri' (1790), he published a calculation of what is now known as the Euler-Mascheroni constant, usually denoted as gamma. Simon Antoine Jean L'Huilier (1750 to 1840) was a Swiss mathematician of French Hugenot descent. He is known for his work in mathematical analysis and topology, and in particular the generalization of Euler's formula for planar graphs. Adrien-Marie Legendre (1752 to 1833) A French mathematician. Legendre polynomials and Legendre transformation are named after him. Legendre is known as the author of 'Éléments de géométrie' which was published in 1794 and was the leading elementary text on the topic for around 100 years. This text greatly rearranged and simplified many of the propositions from Euclid's Elements to create a more effective textbook. Lazar Nicolas Carnot (1753 to 1823) was a French politician, engineer, and mathematician. The Borda-Carnot equation of fluid dynamics and Carnot's theorem in plane geometry are named after him. Captain William Lambton (1753 to 1823) was a British soldier, surveyor, and geographer. He was involved in surveying the boundary between Canada and the United States. He also did surveying in India. Jean Baptiste Meusnier (1754 to 1793) A French mathematician, engineer and Revolutionary general. He is best known for Meusnier's theorem on the curvature of surfaces. He also discovered the helicoid. He worked with Lavoisier on the decomposition of water and the evolution of hydrogen. Jurij Vega (1754 to 1802) was a Slovene mathematician, physicist and artillery officer. Vega published a series of books of logarithm tables. His major work was 'Thesaurus Logarithmorum Completus' (Treasury of all Logarithms) that was first published 1794. Over the years, Vega wrote a four volume textbook 'Vorlesungen über die Mathematik' (Lectures about Mathematics). Volume I appeared in 1782 when he was 28 years old, Volume II in 1784, Volume III in 1788 and Volume IV in 1800. His textbooks also contain interesting tables: for instance, in Volume II one can find closed form expressions for sines of multiples of 3 degrees, written in a form easy to work with. In 1789, Vega achieved a world record when he calculated pi to 140 places, of which the first 126 were correct. Vega had improved John Machin's formula from 1706. Nicolas Fuss (1755 to 1826) was a Swiss mathematician, living most of his life in Russia. He moved to Saint Petersburg to serve as a mathematical assistant to Leonhard Euler from 1773-1783, and remained there until his death. He contributed to spherical trigonometry, differential equations, the optics of microscopes and telescopes, differential geometry, and actuarial science. He also contributed to Euclidean geometry, including the problem of Apollonius. A. Giordano (ca. 1785) "... a Neapolitan lad A. Giordano, who was only 16 but who had shewn marked mathematical ability ..." (need more info and a link) Theodor von Schubert (1758 to 1825) became a tutor of mathematics and astronomy. In 1785, he became an assistant of the Russian Academy of Sciences as a geographer, and by June 1789 he was a full member. In 1803, he became head of the astronomical observatory of the Academy. Wrote some texts on astronomy. Louis Francois Antoine Arbogast (1759 to 1803) was a French mathematician. He wrote on series and the derivatives known by his name. His notion of using discontinuous functions to integrate partial differential equations became important in Cauchy's more rigorous approach to analysis. He conceived the calculus as operational symbols. The formal algebraic manipulation of series investigated by Lagrange and Laplace in the 1770s has been put in the form of operator equalities by Arbogast by 1800. Paolo Ruffini (1765 to 1822) was an Italian mathematician and philosopher. By 1788 he had earned university degrees in philosophy, medicine/surgery, and mathematics. Among his work was an incomplete proof (Abel-Ruffini theorem) that quintic (and higher-order) equations cannot be solved by radicals (1799), and Ruffini's rule which is a quick method for polynomial division. Ruffini also made contributions to group theory in addition to probability and quadrature of the circle. Sylvestre François Lacroix (1765 to 1843) was a French mathematician. He displayed a particular talent for mathematics, calculating the motions of the planets by the age of 14. In 1793 he became examiner of the Artillery Corps, replacing Pierre-Simon Laplace in the post. By 1794, he was aiding his old instructor, Gaspard Monge, in creating material for a course on descriptive geometry. In 1799, he was appointed professor at the École Polytechnique. Lacroix produced most of his texts for the sake of improving his courses. During his career he produced a number of important textbooks in mathematics. Translations of these books into the English language were used in British universities, and the books remained in circulation for nearly 50 years. Thomas Malthus (1766 to 1834), an English-born economist, used arithmetic and geometric progressions to describe the growth of population. Arithmetic progressions and geometric progressions had been known since the time of the Greeks. Joseph Fourier (1768 to 1830) was a French mathematician and physicist. Best known for initiating the investigation of Fourier series and their applications to problems of heat transfer and vibrations. (Any wave form can be made by combining sine and cosine functions.) Jean Robert Argand (1768 to 1822) was a gifted amateur mathematician. In 1806, while managing a bookstore in Paris, he published the idea of geometrical interpretation of complex numbers known as the Argand diagram. Argand is also renowned for delivering a proof of the 'fundamental theorem of algebra' in his 1814 work 'Réflexions sur la nouvelle théorie d'analyse' (Reflections on the new theory of analysis). It was the first complete and rigorous proof of the theorem, and was also the first proof to generalize the fundamental theorem of algebra to include polynomials with complex coefficients. Jean Nicolas Pierre Hachette (1769 to 1834), French mathematician, was born at Mézières, where his father was a bookseller. His labours were chiefly in the field of descriptive geometry and 3 dimensions, with its application to the arts and mechanical engineering. It was left to him to develop the geometry of Monge, and to him also is due in great measure the rapid advancement which France made soon after the establishment of the École Polytechnique in the construction of machinery. Heinrich August Rothe (1773 to 1842) was a German mathematician, a professor of mathematics at Erlangen. The Rothe-Hagen identity, a summation formula for binomial coefficients, appeared in Rothe's 1793 thesis. It is named for him and for the later work of Johann Georg Hagen. The same thesis also included a formula for computing the Taylor series of an inverse function from the Taylor series for the function itself, related to the Lagrange inversion theorem. In the study of permutations, Rothe was the first to define the inverse of a permutation, in 1800. He developed a technique for visualizing permutations now known as a Rothe diagram. Nathaniel Bowditch (1773 to 1838) was an early American mathematician remembered for his work on ocean navigation. He is often credited as the founder of modern maritime navigation. His book 'The New American Practical Navigator', first published in 1802, is still carried on board every commissioned U.S. Naval vessel. Louis Poinsot (1777 to 1859) added two new regular solids to the five Platonic solids and the two 'stellated' regular solids pointed out by Kepler --- for a total of nine. the five Platonic solids (tetrahedron, octahedron, cube, dodecahedron, icosahedron) small stellated dodecahedron - attributed to Kepler and, before Kepler, to artist Paolo Uccello (1397-1475) great stellated dodecahedron - attributed to Kepler and, before Kepler, to artist Wenzel Jamnitzer (1508-1585). great dodecahedron (found by Poinsot) great icosahedron (found by Poinsot) Augustin Cauchy (1789-1857 ; see below) proved that these are the only regular solids. Carl Friedrich Gauss (1777 to 1855) devised a method of constructing a 17-sided polygon using straight edge and compass only. Greek mathematicians had been able to constrocuh polygons of 3 and 5 sides with straight edge and compass only. Gauss wanted a 17-sided polygon on his grave, but the stonemason refused because it would simply look like a circle. Going well beyond this, Gauss showed that a regular n-sided polygon can be so constructed if n is a Fermat prime (2^2^k + 1, for an integer k). The next Fermat primes after 17 are 257 and 65,537. In 1832, F.J. Richelot, who taught mathematics at the University of Konigsberg, showed how to construct a 257-sided polygon. In 1894, J. Hermes wrote out the construction of a regular 65,537 sided polygon. It had taken him 10 years and took up 200 pages. 'Unfortunately, it is likely to contain a mistake.' Gauss proved the 'Fundamental Theorem of Algebra': A polynomial equation of degree n has n roots, in the complex plane. The theorem provides an example of where the theory of complex numbers is more complete than that of the real numbers. Christoph Bernoulli (1782 to 1863) Charles Julien Brianchon (1783 to 1864) was a French mathematician and chemist. He entered into the École Polytechnique in 1804 and studied under Monge, graduating first in his class in 1808, after which he took up a career as a lieutenant in Napoleon's artillery. Later, in 1818, Brianchon became a professor in the Artillery School of the Royal Guard at Vincennes. Brianchon is best known for his proof of Brianchon's theorem (1810). Friedrich Bessel (1784 to 1846) Charles Xavier Thomas de Colmar (1785 to 1870), a Frenchman who served in the French army, in 1820 produced the first really successful commercial calculating machine. (See Schickard, Pascal, and Leibniz above.) By the 1860's, the machine was commercially successful. Under different guises, it continued to be produced for well over 100 years --- to around 1920? or 1960? Claude Louis Marie Henri Navier (1785 to 1836) Jean Victor Poncelet (1788 to 1867) Augustin Cauchy (1789 to 1857) provided rigorous definitions of the concept of continuity of curves and their derivatives. He put the field of 'real and complex analysis' on firm ground. Jean Francois Champollion (1790 to 1832) August Mobius (1790 to 1868) conceived of the Mobius strip, which has just one edge and one surface. George Everest (1790 to 1866) Charles Babbage (1791 to 1871) designed a 'difference engine' and an 'analytic engine' --- motivated by his thought: "I am thinking that these tables [logarithms] might be calculated by steam." The 'difference engine' had a fairly precise task --- to calculate and print out the values of certain functions. Known values of the required function were entered and the machine then calculated other values (thus the 'difference'). The 'analytic engine' was like a modern computer, except that it worked mechanically rather than electronically. It was programmable, in that it could carry out any mathematical task. It could branch and it could loop. The analytic engine was never actually built. Babbage kept changing his mind about what he wanted and quarreled with everyone working with him. The British government withdrew from the project in 1842. The secretary of the Royal Astronomical Society wrote: "We got nothing for our 17,000 pounds but Mr. Babbage's grumblings. We should at least have had a clever toy for our money." Nicolai Lobachevsky (1792 to 1856) developed a system of geometry in which Euclid's 5th postulate (on parallel lines) no longer holds. (See Janos Bolyai.) Ernst Weber (1795 to 1878) Jakob Steiner (1796 to 1863) Gustav Theodor Fechner (1801 to 1887) Janos Bolyai (1802 to 1850) developed a system of geometry in which Euclid's 5th postulate (on parallel lines) no longer holds. (See Nicolai Lobachevsky.) Neils Abel (1802 to 1829), a Norwegian, in 1821, showed that there is no algebraic formula for the roots of the general quintic equation. William Rowan Hamilton (1805 to 1865) was an Irish physicist, astronomer, and mathematician, who made important contributions to classical mechanics, optics, and algebra. His studies of mechanical and optical systems led him to discover new mathematical concepts and techniques. His best known contribution to mathematical physics is the reformulation of Newtonian mechanics, now called Hamiltonian mechanics. This work has proven central to the modern study of classical field theories such as electromagnetism, and to the development of quantum mechanics. In pure mathematics, he is best known as the inventor of quaternions, which can be used to model positions in four dimensions. Thomas Kirkman (1806 to 1895) posed a problem in combinatorics called 'Kirkman's schoolgirl problem'. Sudoku puzzles are an example of these types of combinatoric problem. Joseph Liouville (1809 to 1882), in 1844, constructed the first transcendental numbers --- numbers that were not the solutions of algebraic equations (composed of polynomials). Benjamin Peirce (1809 to 1880) Evariste Galois (1811 to 1832) developed a theory of groups of permutations of solutions to polynomial equations which was used to show that, although quadratic, cubic, and quartic equations had a general solution in the form of an algegraic formula, quintic (and higher) equations do not have such a general solution. 'Group theory' became a major area of mathematics. It has been extened to many other structures besides permutations and is used in nuclear and quantum physics. Johann Gustav Bernoulli (1811 to 1863) Pierre Wantzel (1814 to 1848) - Constructions with straight edge and compass only (like the 3 Greek problems of trisecting the angle, squaring the circle, and doubling the cube) were shown to be equivalent to using the four basic operations of arithmetic ( + - x / ) and taking square roots. The way was then clear to show that the Greek problems were insoluble. If a problem involves a length which cannot be built up from 1 by + , - , x , / , and squre root, then it cannot be constructed and the problem cannot be solved. George Boole (1815 to 1864) set up a system to codify logical argument as a form of algebra. This is used in the design of computer circuitry. Karl Wierstrass (1815 to 1897) is called, by some, "the father of modern analysis". He put calculus on more solid ground by introducing more rigor in definitions and proofs. John Tyndall (1820 to 1893) Hermann Ludwig Helmholz (1821 to 1894) Charles Hermite (1822 to 1901) showed in 1873 that the number 'e' is transcendental. Francis Galton (1822 to 1911) develped the 'correlation coefficient'. Jule Antoine Lissajous (1822 to 1880) Bernhard Riemann (1826 to 1866) - The Riemann Hypothesis is a conjecture about the zeros, in the complex plane, of an infinite series. If this hypothesis can be proved to be true, it will provide the solution to many other unsolved problems. James Clerk Maxwell (1831 to 1879) devised a set of equations summarizing the behavior of electricity and magnetism. Richard Dedekind (1831 to 1916) Alvan Graham Clark (1832 to 1897) John William Strutt (Lord Rayleigh) (1842 to 1919) Arnold Buffum Chace (1845 to 1932) Julius Plucker (1801 to 1868) was a German mathematician and physicist. He made fundamental contributions to the field of analytical geometry and was a pioneer in the investigations of cathode rays that led eventually to the discovery of the electron. He also vastly extended the study of Lamé curves. In 1865, Plücker invented what was known as 'line geometry' in the nineteenth century. In 'projective geometry', Plücker coordinates refer to a set of homogeneous co-ordinates introduced initially to embed the set of lines in three dimensions as a quadric in five dimensions. Felix Klein (1849 to 1925) conceived of the Klein bottle --- a twisted shape that has no inside or outside, and exists only in four dimensions. It can be made by joining two Mobius strips edge to edge, in four dimensions. Oliver Heaviside (1850 to 1925) Sonia Kovalevsky (1850 to 1891) Henri Poincare (1854 to 1912) was a founder of the field of topology. It is said that "Topologists think that a doughnut is the same as a coffee cup." Topologists are not content with objects in two or three dimensions. They extend the definitions of topology into four or more dimensions. Aleksandr Lyapunov (1857 to 1918) - the Central Limit Theorem - the mean of a large enough sample is approximately normal --- that is, if enough data is taken, the normal distribution can be used to calculate probabilities. Max Planck (1858 to 1947) - a quantum physicist Hermann Minkowski (1864 to 1909) was a Lithuanian-German mathematician. He used geometrical methods to solve problems in number theory, mathematical physics, and the theory of relativity. Theodore_Andrea_Cook (1867 to 1928) wrote 'The Curves of Life: Being an Account of Spiral Formations and Their Application to Growth in Nature, to Science and to Art: with the special reference to the manuscripts of Leonardo da Vinci'(1914). According to Cook, Mark Barr, an American mathematician, in about 1909, gave the golden ratio the name of phi, the first Greek letter in the name of Phidias, the Greek sculptor who lived around 450 BC Edmund Georg Hermann Landau (1877 to 1938) was a German mathematician who worked in the fields of number theory and complex analysis. In 1903, Landau gave a much simpler proof than was then known of the prime number theorem and later presented the first systematic treatment of analytic number theory in the 'Handbuch der Lehre von der Verteilung der Primzahlen'. Albert Einstein (1879 to 1955) - 'nuf said. Oswald Veblen (1880 to 1960) was an American mathematician, geometer and topologist, whose work found application in atomic physics and the theory of relativity. He proved the Jordan curve theorem in 1905. The Jordan Curve Theorem states that a continuous closed curve cuts the plane into two distinct parts. There are so many 'pathological' curves, such as the snowflake curve and space-filling curves, that a complete proof is very tricky. Lipot Fejer (1880 to 1959) was a Hungarian mathematician. In 1911, Fejér was appointed to the chair of mathematics at the University of Budapest and he held that post until his death. He was the thesis advisor of mathematicians such as John von Neumann, Paul Erdos, George Pólya and Pál Turán. Fejér's research concentrated on harmonic analysis and, in particular, Fourier series. Fejér collaborated to produce important papers, such as a major work with Frigyes Riesz in 1922 on conformal mappings (specifically, a short proof of the Riemann mapping theorem). Emmy Noether (1882 to 1935) was an influential German mathematician known for her groundbreaking contributions to abstract algebra and theoretical physics. Described by Pavel Alexandrov, Albert Einstein, Jean Dieudonné, Hermann Weyl, Norbert Wiener and others as the most important woman in the history of mathematics, she revolutionized the theories of rings, fields, and algebras. In physics, Noether's theorem explains the fundamental connection between symmetry and conservation laws. Aleksandr Friedmann (1888 to 1925) was a Russian and Soviet physicist and mathematician. He is best known for his pioneering theory that the universe is expanding, governed by a set of equations he developed now known as the Friedmann equations. Karl Menger (1902 to 1985) was an American mathematician. He is credited with Menger's theorem. He worked on mathematics of algebras, algebra of geometries, curve and dimension theory, etc. Moreover, he contributed to game theory and social sciences. He has been associated with 'the traveling salesman problem' : A traveling salesman must visit a number of cities. What is the most efficient route? Oskar Morgenstern (1902 to 1977) was a German-born economist. In collaboration with mathematician John von Neumann, he founded the mathematical field of 'game theory' and its application to economics. John von Neumann (1903 to 1957) was a Hungarian pure and applied mathematician. He made contributions in many areas of mathematics and physics, including game theory. He worked on the Manhattan project, which was set up in 1942 to develop atomic bombs. George Stibitz (1904 to 1995), a Bell Labs researcher, in 1937 built a machine, on his kitchen table, that did binary arithmetic. He is known for his work in the 1930s and 1940s on the realization of Boolean logic digital circuits using electromechanical relays as the switching element. (See Claude Shannon, below.) George Gamow (1904 to 1968) was a theoretical physicist and cosmologist - notably an early advocate and developer of Lemaître's Big Bang theory. He discovered a theoretical explanation of alpha decay via quantum tunneling, and worked on radioactive decay of the atomic nucleus, star formation, stellar nucleosynthesis and Big Bang nucleosynthesis (which he collectively called nucleocosmogenesis), and molecular genetics. In his middle and late career, Gamow focused more on teaching, and became well known as an author of popular books on science, including 'One Two Three ... Infinity'. Marian Rejewski (1905 to 1980) a Polish mathematician who succeeded in breaking the basic Enigma code of the Germans near the start of world war II. Tommy Flowers (1905 to 1998), a telecommunications and electronics expert, using his experience of the British telephone system, proposed the 'Colossus' electronic computer for decoding top-secret German messages. Albert W. Tucker (1905 to 1995) dealt with the prisoner's dilemma, the most famous example in game theory: Two suspects are arrested. Should each one stay silent or confess to the crime? Alexandr Osipovich Gelfond (1906 to 1968) was a Soviet mathematician. His most famous result is the Gelfond theorem: If alpha and beta are algebraic numbers (with alpha not equal 0 or 1), and if beta is not a real rational number, then any value of alpha-to-the-beta is a transcendental number. Before Gelfond's work, only a few numbers such as e and pi were known to be transcendental. After his work, an infinite number of transcendentals could be easily obtained. Some of them are named in Gelfond's honor: 2^sqrt(2) is known as the Gelfond-Schneider constant, and e^pi is known as Gelfond's constant. John Mauchly (1907 to 1980) helped develop ENIAC, the first, general-purpose electronic computer. (See Eckert below.) Claude Shannon (1916 to 2001) in 1937 showed how to design circuits for binary arithmetic. Charles Frederick Mosteller (1916 to 2006) was one of the most eminent statisticians of the 20th century. He was the founding chairman of Harvard's statistics department, from 1957 to 1971. Mosteller wrote over 50 books and over 350 papers, with over 200 coauthors. He was an avid fan of the Boston Red Sox and attended the games in the run up to the World Series. He conducted perhaps the first academic investigation of baseball after his favorite team, the Boston Red Sox, lost the 1946 World Series. He has been mentioned with 'the Secretary Problem' - choosing the best secretary from a pool of candidates. Edward Norton Lorentz (1917 to 2008) was an American mathematician and meteorologist, and a pioneer of chaos theory. He discovered the strange attractor notion and coined the term 'butterfly effect'. J. Presper Eckert (1919 to 1995) helped develop ENIAC, the first, general-purpose electronic computer. (See Mauchly above.) Rene Thom (1923 to 2002) - Catastrophe theory deals with slight changes that can lead to great changes. Thom sought to find a mathematical way to analyze catastrophic changes. Roger Penrose (1931 to ?), an English physicist, invented several 'tesselations' of the plane, built up from two quadrilaterals known as 'kites' and 'darts', due to the shape of those quadrilaterals. These tesselations are not periodic: they do not repeat themselves and any two parts of the tesselation are essentially different from each other. Robert May (1938 to ?) is an Australian scientist who studied chemical engineering and theoretical physics (B.Sc. 1956) and received a Ph.D. in theoretical physics in 1959. He has provided a model for population growth that takes limited resources into account. Reference: His 1976 paper "Simple mathematical models with very complicated dynamics".

Bottom of this Physics History - A Timeline page. To return to a previously visited web page location, click on the Back button of your web browser, a sufficient number of times. OR, use the History-list option of your web browser. OR ... < Go to Start of Timeline, above. > < Go to Top of Page, above. > Page history: Page was created 2017 Mar 10. Page was changed 2019 Mar 11. (Added css and javascript to try to handle text-size for smartphones, esp. in portrait orientation.) Page was changed 2019 Oct 14. (Specified some image widths in accordance with the width of the browser window.) NOTE: This web site may go dead around 2020 to 2030. Anyone who wishes to put a copy of this page on their own web site is welcome to do so. And you are welcome to change and add to your copy.